International Journal of Advanced Research in Electrical, Electronics and Instrumentation Engineering

International Journal of Advanced Research in Electrical, Electronics and Instrumentation Engineering

Menu

ISSN ONLINE(2278-8875) PRINT (2320-3765)

All submissions of the EM system will be redirected to Online Manuscript Submission System. Authors are requested to submit articles directly to Online Manuscript Submission System of respective journal.

Comparative Analysis of Types of Shunt Hybrid Power Filter Using Instantaneous Power Theory

Sona.P1, K.Radhakrishnan2, Dr. Bos Mathew Jos3
  1. P.G. Student, Mar Athanasius College of Engineering, Kothamangalam, Kerala, India 1
  2. Professor, Mar Athanasius College of Engineering, Kothamangalam, Kerala, India2
  3. Assistant Professor, Mar Athanasius College of Engineering, Kothamangalam, Kerala, India3
Related article at Pubmed, Scholar Google

Visit for more related articles at International Journal of Advanced Research in Electrical, Electronics and Instrumentation Engineering

International Journal of Advanced Research in Electrical, Electronics and Instrumentation Engineering

Abstract

The use of nonlinear load led to the injection of harmonics into the power system. In this paper, the performance of two topologies of shunt hybrid power filters for compensating voltage and current harmonics in the distributed power generation (DPG) system has been compared. The control algorithm used in this paper is the p-q theory for the harmonics compensation. The performance of these topologies are verified through MATLAB/Simulink for RL load. The THD of the power system is reduced to minimum tolerance level (< 5%) by the use of both hybrid filters. The THD of the power system is reduced from 30.26% to 5.05% and 4.57 % with the use of hybrid filter with parallel connected active and passive filters and with series connected active and passive filter respectively

Keywords
Shunt hybrid power filter, Instantaneous power theory, harmonics, THD

INTRODUCTION
A good power quality is an important factor for the reliable operation of electrical loads. In recent years, high penetration of nonlinear loads such as compact fluorescent lamps, light-emitting diode lamps and switching mode power suppliers give rise to serious challenges in power quality for distribution systems. Harmonic distortion produced by nonlinear loads causes several problems such as increased power losses in customer equipment, power transformers and power lines, flicker, shorter life of organic insulation etc. The installation of DPG to the distribution system may have a significant impact on power quality. This impact may be positive or negative. It depends on the distribution system and DPG unit characteristics. Positive impacts includes improved reliability, voltage support, reduction of transmission and distribution losses. However, in some cases inverter-based DPG units can increase the current and voltage distortions in the system to which they are connected. Between the different technical options available to power quality improving active power filters (APF) have proved to be an important alternative to compensate for current and voltage disturbances in power distribution systems. In recent years active power filters have been widely investigated for the compensation of harmonic currents.
The shunt active filter for a single-phase DPG system is considered in [2]. However, in many cases installation of pure active filters is not cost effective solution because their ratings are close to load. The hybrid power filters (HPF) seem to be more attractive solution for current and voltage disturbance mitigation

SYSTEM CONFIGURATION
The two topologies of HPF comprising of a series combination of active and passive filters and a parallel combination of active and passive filters are shown in the fig. 2 and 3 respectively, connected to the power system feeding the nonlinear load. The active filter in both topologies is a voltage source inverter (VSI) with a DC-link capacitance (Cdc) at the DC side and a filter inductor (Lc) to attenuate the ripple of the converter current caused by the switching of the converter[8]. Two single tuned low pass filter tuned to 7thharmonics are used with the activefilter.

CONTROL STRATEGY
The control strategy proposed here aims at making the compensated line current to be sinusoidal and balanced. Therefore the objectives include a sinusoidal reference current calculation and a current control technique for generating switching pulses to the VSI for a sinusoidal and a balanced line current.The control strategy used here is based on the p-q theory introduced by Akagi [3] and expanded to three-phase four-wire systems by Aredes. It applies an algebraic transformation (Clarke transform) of the three-phase system voltages and load currents in the a-b-c coordinates to the α-��-0 coordinates. After the transformation,[11] the p-q theory components are achieved by the expressions (3)-(4), where p is the instantaneous real power and q is the instantaneous imaginary power.
The instantaneous power p-q is based on time domain transformation from a,b,c phases to three orthogonal axes i.e. α-��-0 transformation. This can be applied to three-phase system with or without neutral. The p-q theory uses α-��-0 or Clarke transformation given by eq. (1) & (2). Ifvo and io are neglected, instantaneous power vectors are given as eq. (3) & (4). The (α-p,q) (��-p,q) components can be defined in eq. (5).
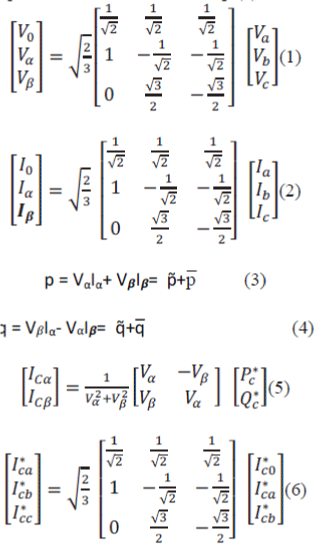image
The p and q components of voltage, current, active power can be separated by using above equations, which further decides the compensation methodology to be applied to a particular system. The p-q theory has selecting the component independently. This can be understood clearly from the block diagram shown in fig. 4. The α, β components of voltage and current are used for calculating instantaneous real and reactive power. Among this the harmonic real power component and the entire reactive power are used for the compensation current calculation. This compensation current is injected to the power system to nullify the harmonic components in the system.

A. Passive Filter Design
At the harmonic frequency n , the filter impedance
image
Where XFL and XFC are filter impedances due to inductance and capacitance Therefore the current injected into the line is, neglecting resistance
image
Here all the quantities are referred to the same voltage level
image
then the required value of the capacitor can be found out for a set limit η[12].
The passive filter used in the topology is tuned for eliminating 7 th harmonics. The passive filter parameters are L= 0.4μF and C= 3.3Mh

SIMULATION DIAGRAM AND RESULTS
The two topologies of Hybrid filters are simulated using MATLAB/ SIMULINK. The simulation of p-q theory is carried out for the compensating current calculation of the three-phase voltage source supplying thyristor rectifier with RL load(18 Ω, 10mH) . The p-q theory provides complete analysis of different power components for analysis and compensation. The time-domain calculation allows deciding about the exact instant and amount of compensation to be provided for elimination. The rms value of the fundamental component being 400V. The active filter parameters are L=3.35mH, R=0.4 ohms, DC link capacitance = 40mF , DC link reference voltage Vdc.ref=800V. The passive filter is tuned for eliminating 7th harmonics and the parameters are L= 0.4μF and C= 3.3mH.
Fig. 9, 10, & 11 shows the simulation results of source currents, compensated currents , real power and reactive power of power system without compensation, with series combination of passive and active filters, with parallel combination of passive and active filters respectively.
The THD of the power system is reduced from 30.26% to 5.05% and 4.57 % with the use of hybrid filter with parallel connected active and passive filters and with series connected active and passive filter respectively. The FFT analysis of source current without compensation, with hybrid filter having parallel connected active and passive filters and series connected active and passive filter are shown below in fig. 12

CONCLUSION
Two types of hybrid filter topology with a series and parallel combination of active and passive filters are simulated for a three phase system. It replaces an already existing active filter to improve the harmonic compensation, power factor etc. The passive filter performance might have effected due to changes in the system parameters. This topology helps in improving the performance of passive filter and at the same time, the active filter can be used at a lower rating. Use of passive filters in parallel with an active filter reduces the load distortion bandwidth to be compensated by the active filter. The performance of the control strategy used in this paper is simple and effectively compensates the load generated harmonics and nullifies the effect of the source voltage harmonics in the line. It reshape the distorted waves to practically sinusoidal waves. The FFT analysis is done and THD of the power system is found to be reduced from 30.26% to 5.05% and 4.57 % with the use of hybrid filter with parallel connected active and passive filters and with series connected active and passive filter respectively. In the future this can be interfaced with the renewable energy sources so that it will work as compensator as well as inverter.

Figures at a glance
Figure 1 Figure 2 Figure 3 Figure 4
Figure 1 Figure 2 Figure 3 Figure 4
Figure 5 Figure 6 Figure 7 Figure 8
Figure 5 Figure 6 Figure 7 Figure 8
Figure 9 Figure 10 Figure 11 Figure 12
Figure 9 Figure 10 Figure 11 Figure 12
 

References
  1. C.N Srivatsav, Dr.Pramod Agarwal ,ChendikaVenkataAvinash ‘Types of hybrid power filter and their comparative analysis with distorted source voltage using MATLAB”2014 ICAECT
  2. Vasundhara Mahajan, Pramod Agarwal, Hari Om Gupta “Simulation Of Shunt Active Filter Using Instantaneous Power Theory” 2012 Power India Conference IEEE fifth, Murthal
  3. H. Akagi, Y. Kanazawa, A. Nabae, “Generalized Theory of the Instantaneous Reactive Power in Three-Phase ircuits”, IPEC'83 - Int. Power Electronics Conf., Tokyo, Japan, 1983, pp. 1375-1386.
  4. Singh B., Verma V, “An indirect current control of hybrid power filter for varying loads”, IEEE transaction on power delivery, 21, no.1 , jan 2006
  5. Furtado, P.C.S. Rodrigues, Barbosa, “Two phase three wire active power filter using single phase p-q theory” Power Electronics Conference 2013(COBEF) 2013 Brazilian.
  6. Taruna Jain, Shailendra Jain, Ganga Agnihotri, “ Comparison of Topologies of Hybrid Active Power Filter”, International conference on Information and communication technology in Electrical Sciences,Dec.2007, pp.503-509
  7. F. Blaabjerg, R. Teodorescu, M .Liserre., A . Timbus, “Overview of control and grid synchronization for distributed power generation systems,”IEEE Trans. on Industrial Electronics, vol. 53, pp. 13981409, Oct.2006.
  8. Narayan Prasad Gupta., Preeti Gupta, and Dr.DeepikaMasand “Performance Evaluation of Hybrid Active Power Filter” International Conference on Communication Systemand Network Technologies IEEE 2012.
  9. J. Rocabert, A. Luna, F. Blaabjerg, P. Rodrigues, “Control of power converters in AC microgrids,” IEEE Trans. on Power Electronics, vol. 27, pp. 4734-4749, Nov. 2012.
  10. M. Cirrincione, M. Pucci, G.Vitale, “A single-phase DG generation unit with shunt active power filter capability by adaptive neural filtering,” IEEE Trans. on Industrial Electronics, vol. 55, pp. 2093-2110, May 2008.
  11. A.E. Fitzgerald, Charles Kingsley,Jr, Stephen D. Umans “Electrical Machinery” 6 th edition Tata McGraw Hill Pvt Ltd
  12. R.D.Begamudre “Electromechanical Energy Conversion with Dyanamics of Machines” 3 th edition Tata McGraw Hill Pvt Ltd