ISSN ONLINE(2319-8753)PRINT(2347-6710)
ISSN ONLINE(2319-8753)PRINT(2347-6710)
A.Nellai Murugan1, G.Victor Emmanuel2
|
| Related article at Pubmed, Scholar Google |
Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology
In this paper, we introduce the degree equitable domination number in graphs. Some interesting relationships are identified between domination and degree equitable domination and independent domination. It is also shown that any positive integers with are realizable as the domination number, degree equitable domination number and independent domination number of a graph. We also investigate the degree equitable domination in the corona of graphs.
Keywords |
| Degree equitable dominating set, degree equitable domination number, |
I. INTRODUCTION |
| The concept of domination in graphs evolved from a chess board problem known as the Queen problem- to find the minimum number of queens needed on an 8x8 chess board such that each square is either occupied or attacked by a queen. C.Berge[3] in 1958 and 1962 and O.Ore[8] in 1962 started the formal study on the theory of dominating sets. Thereafter several studies have been dedicated in obtaining variations of the concept. The authors in [7] listed over 1200 papers related to domination in graphs in over 75 variation. |
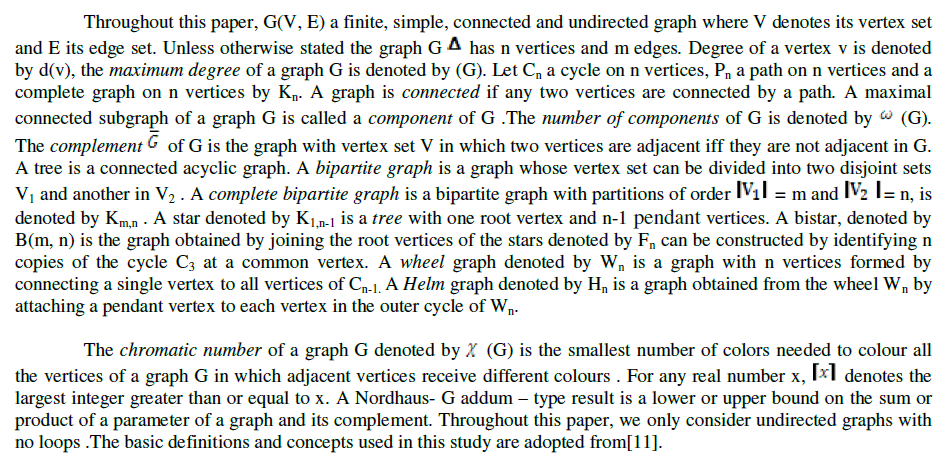 |
 |
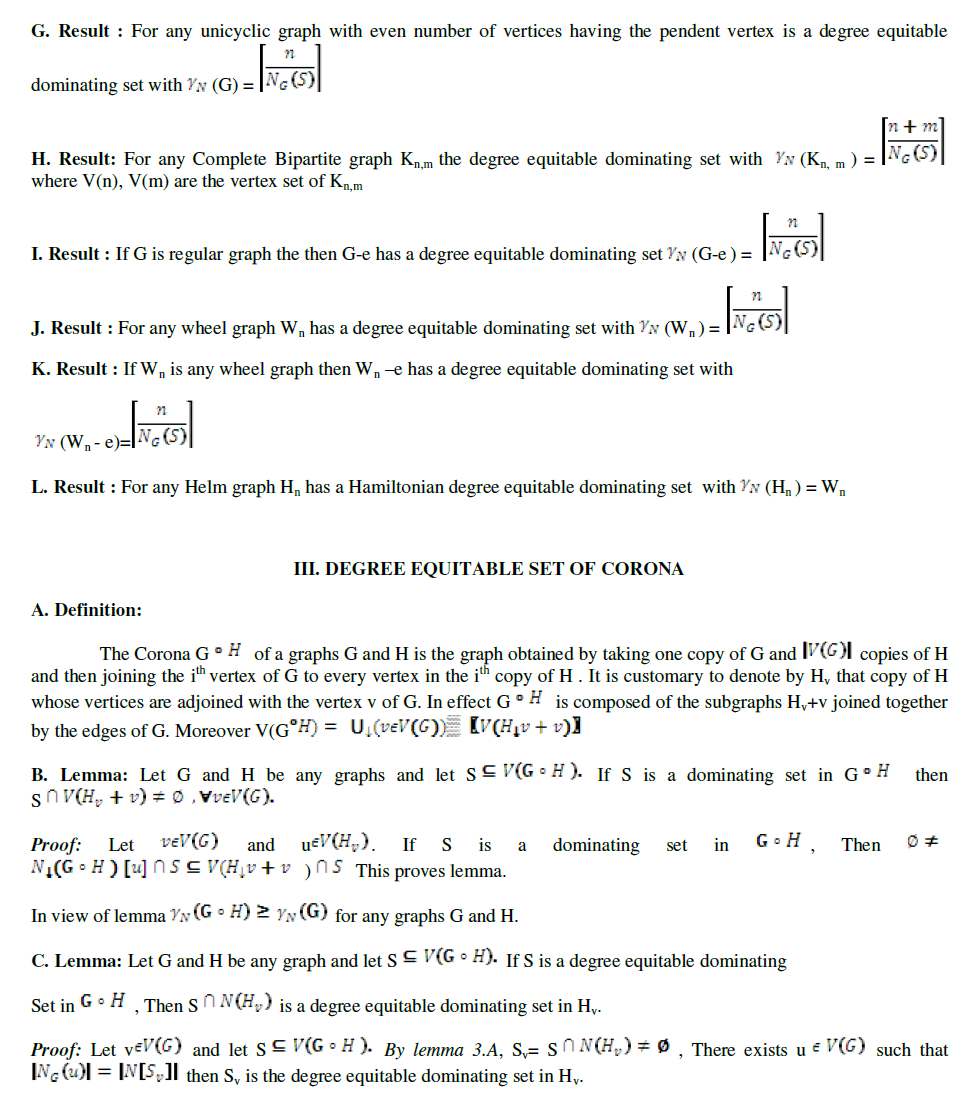 |
 |
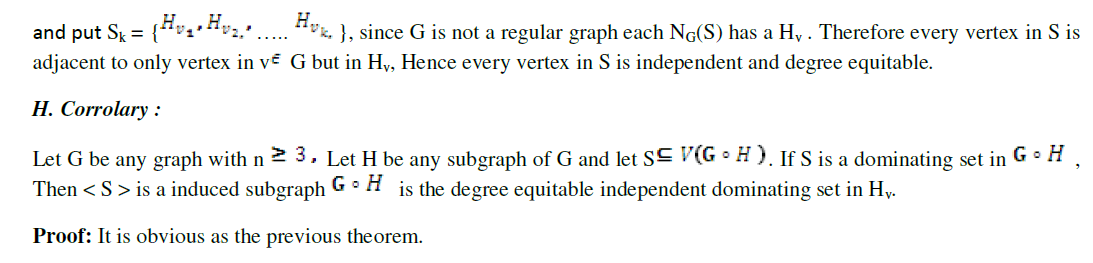 |
References |
|