International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Menu

ISSN ONLINE(2319-8753)PRINT(2347-6710)

All submissions of the EM system will be redirected to Online Manuscript Submission System. Authors are requested to submit articles directly to Online Manuscript Submission System of respective journal.

Novel High-Performance High-Valency Ling Adders

P.Santhosha 1, M.Rajeswara Rao 2
  1. P.G. Student, Department of ECE, SIETK, Andhra Pradesh, India
  2. Assistant Professor, Department of ECE, SIETK, Andhra Pradesh, India
Related article at Pubmed, Scholar Google

Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Abstract

Parallel prefix adders are used for economical VLSI implementation of binary variety additions. The proposed Ling design offers a quicker carry computation stage compared to the standard parallel prefix adders by projecting a replacement methodology to solve Ling adders, which helps to cut the complexity and also the delay of the adder. This paper discusses the look and implementation details for such lower complex, quick parallel prefix adders supported Ling theory of resolving. Specifically, valency or node indicates number of inputs given to a particular node in a carry tree. The proposed ling adder shows that the high-valency Ling adders have superior space area and delay characteristics over antecedent reportable adders for identical input size. Moreover, our 20-bit high valency adder features a higher space and delay measuring than the antecedent used 16-bit adders.

Keywords
Parallel prefix adders, Area, Delay, Valency and Node.

INTRODUCTION
One of the elemental operations in electronic circuits is Binary Addition. Several fashionable circuits contain many adder units for applications like arithmetic logic unit; thus, there's a substantial interest to style less advanced and better speed and adder architectures. Within the past few decades, numerous architectures of adders are planned to optimize the delay of the adder, examples embrace, carry-look ahead, ripple carry and parallel prefix adder. The parallel prefix adder is one in all the foremost in style architectures and offers sensible compromise among power, space and speed. This sort of adder implements a logic performs to see whether or not every bit position kills the carry or propagates it or generates it. Then these generate and propagate/not kill functions are hierarchically combined to cipher the carry into every bit position forming a carry tree. The ultimate stage computes add at as position using exclusive or (XOR) gates [1]. The rest of paper is structured as follows. Section2 gives summary of distinction between parallel prefix adders and others, and section3 presents the structure of parallel prefix adders. Section4 describes types of parallel prefix adders, Section 6 describes proposed ling adder. Section6 gives the simulation results and section7 provides conclusion of the paper.

RELATED WORK
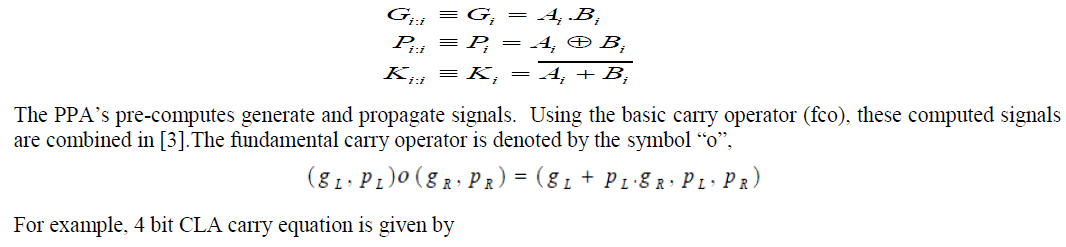
In order to calculate the carries earlier with less delay and less complexness we have a tendency to use parallel prefix adders [2]. Three operations are often outlined to explain standing of carry (a) Propagate: Previous carry is propagated to next bit (b) Generate: Generate a carry bit (c) Kill: Kill the previous carry
image
image
image
image
image
image
image

CONCLUSION
Ling factorization may be recursively applied any to any or all stages during a carry computation tree of an adder. This factorization reduces the complexness of the carry path that is mostly the vital path of the adder, creating it quicker. This makes another ways a lot of advanced, however if the complexness is correctly balanced the ensuing adder will work quicker. Plan of mixing a lot of simplified logic during a cluster while not buffers in between so drives another stage of logic, which is high valency implementation of carry stages. Because of using valency-4, 16-bit adders resulted in similar speed as that of the standard adders, enforced use of Ling factorization at the primary level, however space improvement is sizable. Using valency-5, 20-bit adders are higher in terms of each speed and space than the standard adders. Hence, it's shown that top valency Ling adders have superior space and delay characteristics over existing adders.

References
  1. A. Weinberger and J. L. Smith, “A logic for high speed addition”, National Bureau of Standards, Circulation 591, pp. 3-12, 1958.
     
  2. T. Lynch and E. Swartzlander, “A spanning tree carry look ahead adder”, IEEE Trans. on Computers, vol. 41, no. 8, pp. 931-939, 1992.
     
  3. G. Yang, S. Jung, K. Baek, S. Kim, S. Kim, S. Kang, “A 32-bit carry lookahead adder using dual-path all-N logic”, IEEE Trans. on VLSI Systems, vol. 13, no. 8, pp. 992-996, 2005.
     
  4. S. Hauck, M. Hosler and T. Fry, “High performance carry chains for FPGAs”, IEEE Trans. on VLSI Systems, vol. 8, no. 2, pp. 138-147, 2000.
     
  5. C. Huang, J. Wang, C. Yeh and C. Fang, “The CMOS carry-forward adders”, IEEE Journal of Solid-State Circuits, vol. 39, no. 2, pp. 327- 336, 2004.
     
  6. R.E. Ladner and M.J. Fischer, “Parallel prefix computation”, Journal of ACM, vol. 27, no.4, pp.831-838, Oct. 1980.
     
  7. S. Knowles, “A family of adders”, Proc. 14th IEEE Symp. On Computer Arithmetic, pp.30-34, 1999.
     
  8. P.M. Kogge and H.S. Stone, “A parallel algorithm for efficient solution of a general class of recurrence equations”, IEEE Trans. On Computers, vol. C-22, no. 8, pp.786-793, Aug. 1973.
     
  9. H. Ling, “High speed binary adder”, IBM Journal of Research and Development, vol. 25, no. 3, pp. 156-166, 1981.
     
  10. S. Das and S. P. Khatri, “A novel hybrid parallel-prefix adder architecture with efficient timing-area characteristic”, IEEE Trans. On VLSI Systems, vol. 16, no. 3, pp. 326-331, 2008.