International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Menu

ISSN ONLINE(2319-8753)PRINT(2347-6710)

All submissions of the EM system will be redirected to Online Manuscript Submission System. Authors are requested to submit articles directly to Online Manuscript Submission System of respective journal.

Common Fixed Point Theorems for Pair of Generalized Multi-valued Mappings in Cone Metric Spaces

S. K. Tiwari1, R. P. Dubey2
  1. Asst. Professor, Department of Mathematics, Dr. C. V. Raman University, Bilaspur (C.G.) India
  2. Professor, Department of Mathematics, Dr. C. V. Raman University, Bilaspur(C.G.), India
Related article at Pubmed, Scholar Google

Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Abstract

In This paper, we generalize and obtain common fixed point theorems for a pair of mappings satisfying generalized multi-valued type contractive condition in the setting of cone metric spaces with normal constant 1.Our results generalize the recent results of varies authors

Keywords
Cone metric spaces, common fixed point, multi-valued mapping, contractive Condition, normal cone.

I. INTRODUCTION
In 1970, Covitz Nadler’s (see [6]) gave the following results “Multi-valued contraction mappings generalized metric spaces” using this result. H. E. Kunze et, al (see [3]) introduce an iterative method involving projections that guarantees convergence, from any starting point to a point the set of all fixed points of a multifunction operator T. The results [3] were generalized by Dubey [16]. Especially, Nadler’s. Jr. [7] gave a generalization of Banach’s contraction principle to the case of set-valued maps in metric spaces. Recently, Huang and Zhang [1] introduced the concept of cone metric space by replacing the set of real numbers by an ordered Banach space and obtain some fixed point theorems for mappings satisfying different contractive conditions. Subsequently, the results [1] were generalized and studied the existence of common fixed points of a pair of self mappings satisfying a contractive type condition in the frame work of normal cone metric spaces, see for instance [2], [4], [5],[9] and [11]. The authors [10, 14] introduced the concept of multi-valued contractions in cone metric spaces and using the notion of normal cones, obtained fixed point theorems for such mappings. As we know, most of known cones are normal with normal constant . Further, the author [12] and [13] proved two results, fixed points and common fixed points of multifunction on cone metric spaces. These results also generalized by Dubey and Narayan [17].In this paper, we prove common fixed point theorems for pair of multi-valued maps in cone metric spaces with normal constant K=1, which generalize and extend the results of [1], [8] and [15].

II. PRELIMINARY NOTES
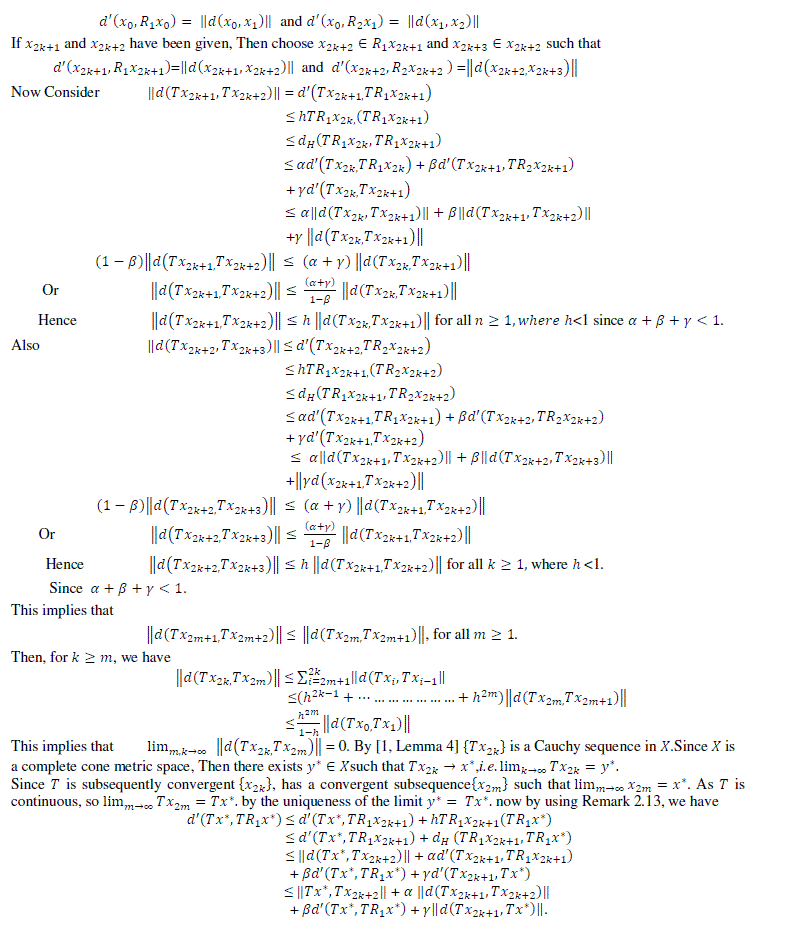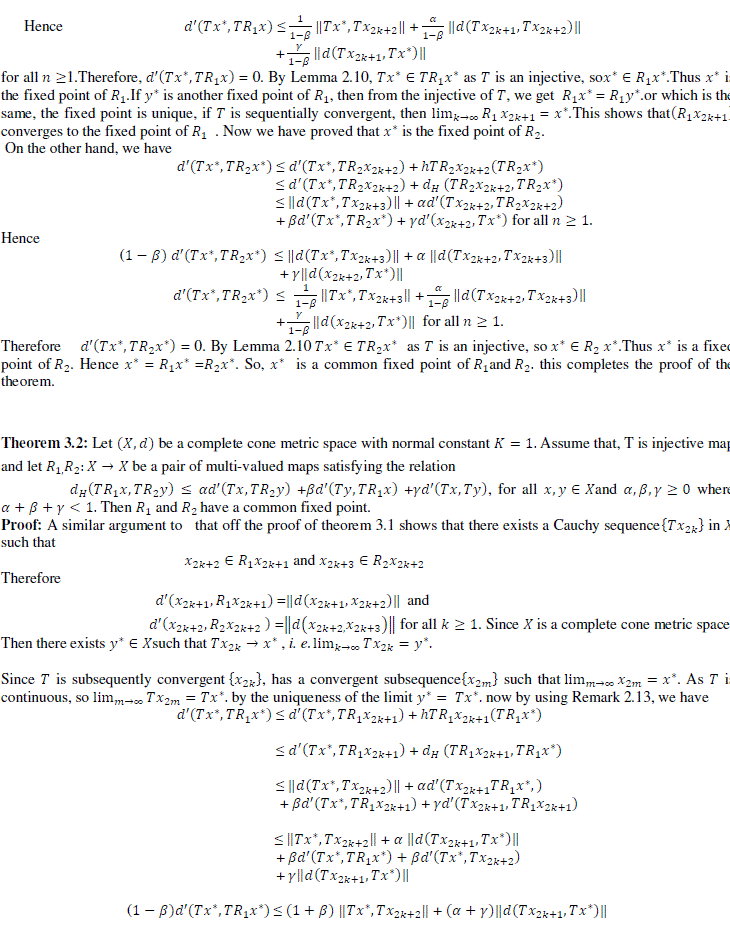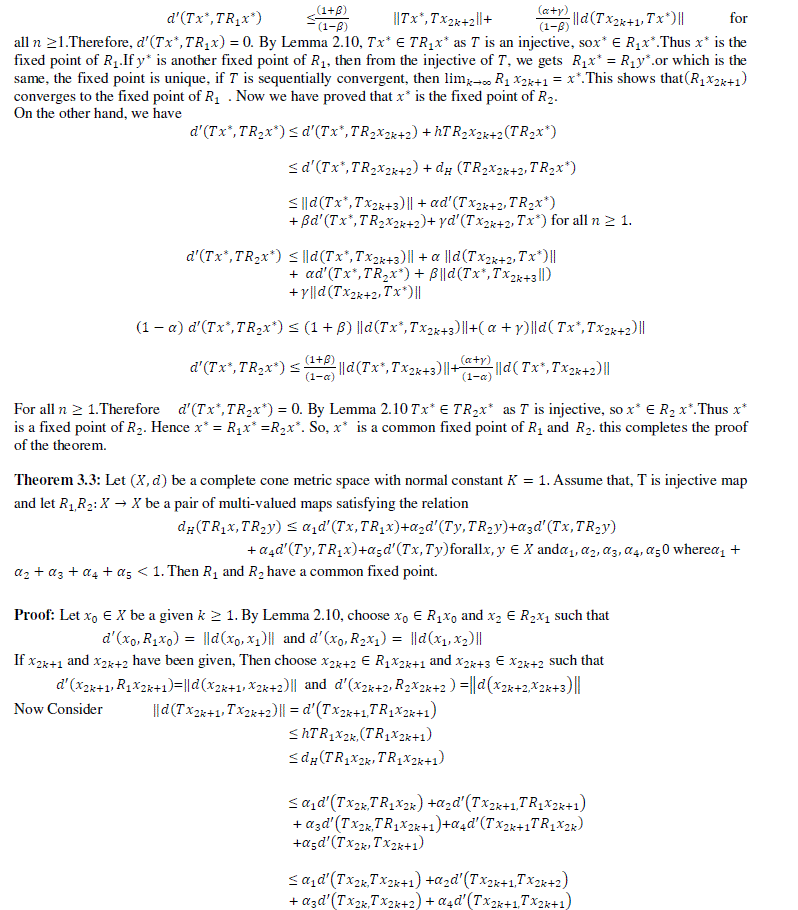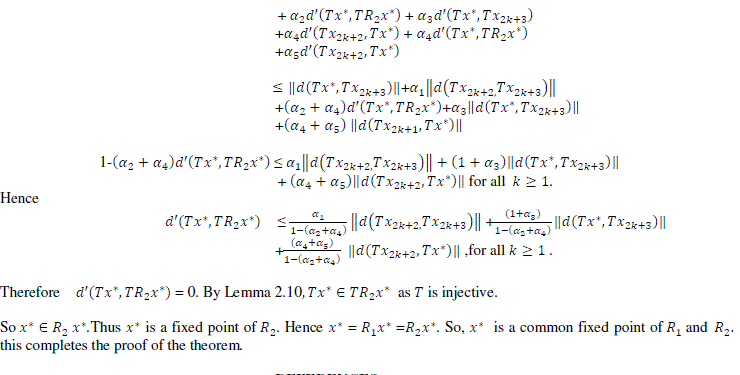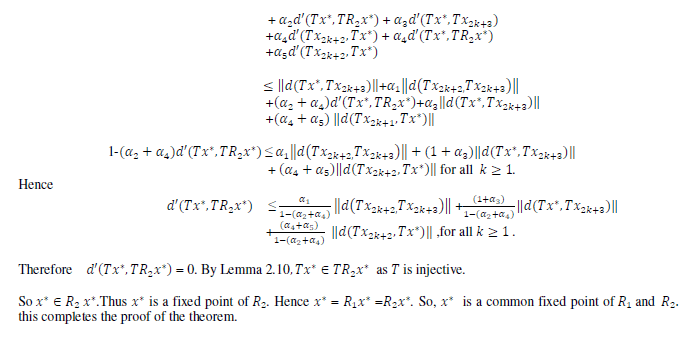image
image
image
image
image
image
image
image
image

References
  1. Huang, L.G and Zhang, X. “Cone metric spaces and fixed point theorems of contractive Mappings”, J. Math. Anal. Appl., 332 (2007), 1468- 1476.
  2. Abbas, M. and Jungck, G. “Common fixed point results for non-commuting mapping without continuity in Cone metric spaces”, J. Math. Anal. Appl., 341 (2008), 416-420.
  3. Kunze, H. E., Torre, D. La. and Vrscay, E. R. “Contraction multifunction, fixed point Inclusions Iterated multifunction systems”, J. Math.Anal. Appl. 330 (2007), 159- 173.
  4. Abbas, M. and Rhoades, B. E. “Fixed and periodic point results in cone metric spaces Appl. Math. Lett, 22 (2009), 511-515.
  5. Abbas, M., Rhoades, B. E. and Nazir, T. “Common fixed points of generalized Contractive Multi-valued Mappings in cone metric spaces”, Math. Commun. 14(2) (2009) 365-378.
  6. Covitz, H. and Nadler, S. B. “Multi-valued contraction mapping in generalized metric Spaces”, Isarael J.Math., 8(1970), 5-11.
  7. Nadler, S.B. Jr. “Multi-valued contraction mappings” Pacific J. Math., 30(2) (1969), 475-488.
  8. Dimri, R. C. Singh A. and Bhatt, S. “Common fixed point theorems for multivalued maps in cone metric spaces”, Int. Math. Forum, 5 (46) (2010), 2271-2278.
  9. Radanovich, S. and Rhoades, B. E. “Fixed point theorem for two non-self mappings in cone metric spaces”, Compute. Math. Appl., 57 (2009), 1701-1707.
  10. Ray, B. “On simultaneous fixed point of multi-valued maps”. Monatshefte. Fur Mathematic, 76 (1972), 448 - 454.
  11. Rezapour, S. and Hamlbarani, R. “Some notes on the paper cone metric spaces and fixed point theorems of Contractive mapping”, J. Math. Anal. Appl., 345 (2008), 719-724.
  12. Rezapour, S. and Hamlbarani, R. Fixed point of multifunction’s on cone metric spaces, Numerical function-al Analysis and Optimization, 30(7) (2009), 825-832.
  13. Rezapour, S.“Two result about common fixed points of multifunction’s on cone metric spaces”, Mathematical proceedings of the Royal Irish Academy, 109 A (2) (2009), 155- 161.
  14. Wardowski, D. “End points and fixed point of set valued contractions on cone metric Spaces”, NonlinearAnalysis: Theory, Methods Applications, 71 (2009), 512-516.
  15. Krishnakumar, R. and Marudai, M. “Generalization of fixed point theorem in cone Metric spaces”, Int. J.Of Math. Analysis, 5(2011) 507-512.
  16. Dubey, A.K. and Narayan, A. “Common fixed point theorems for contractive Multifunction’s”, Int. J. OfPure and Appl. Math., 82(2) (2013) 213 -220.
  17. Dubey, A.K., Narayan, A. and Dubey, R. P. “Common fixed point of multifunction Theorems in coneMetric spaces”, Int. J. Of Appl Mathematical Research, 2(1) (2013), 128-133.
  18. Amini-Harandi, A. “A fixed point results in strictly convex Banach spaces”, Acta Mathematica. Hungerica,15 (1-2) (2004), 139-143.