Jarratt Second Order Method System of Nonlinear Equations

S; eep Singh

Jarratt Second Order Method System of Nonlinear Equations

Sandeep Singh
Dept. of Mathematics, D. A. V. College, Chandigarh, India

Related article at Pubmed, Scholar Google

Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology

Abstract

In this paper, we present scond -order Jarrat method for computing zeros of system of nonlinear equations numerically. In this paper, we extending the idea of the proposed families of Jarrat method to system of nonlinear equations .It is proved that the above said families have second order of convergence. Several numerical examples are also given to illustrate the efficiency and the performance of the presented families.

Keywords

System of Nonlinear equations, Optimal Order of Convergence, Halley's method, Schroder's method, Jarrat method.

INTRODUCTION

Due to the fact that systems of nonlinear equations arise frequently in science and engineering they have attracted researcher's interest. For example, nonlinear systems of equations, after the necessary processing step of implicit discretization, are solved by finding the solutions of systems of equations. We consider here the problem of finding a real zero, x*= (x*1, x*2…….; x*n)T, of a system of non linear equations

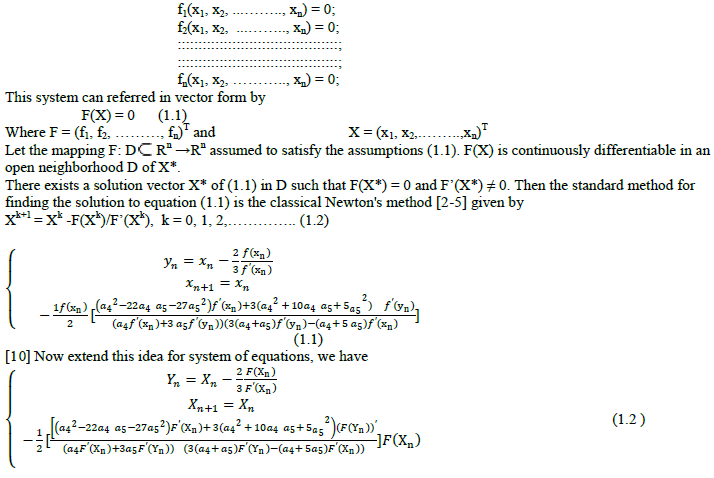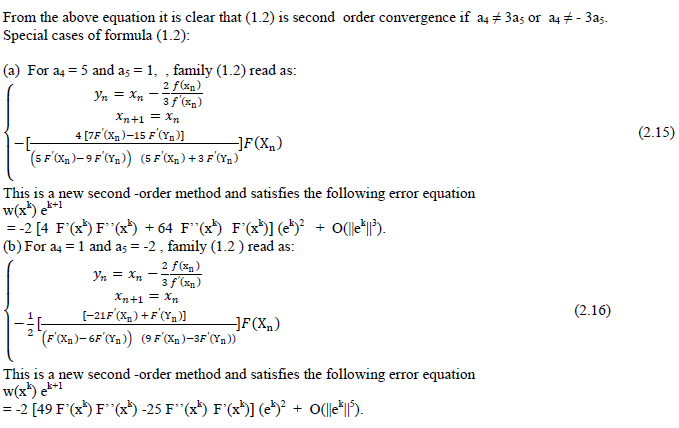NUMERICAL RESULTS

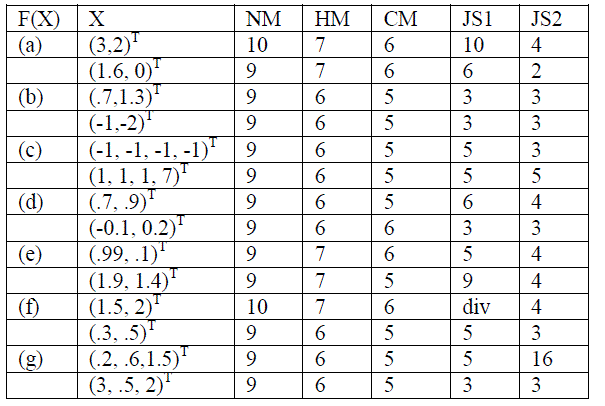
In this section, we shall check the performance of the present formula JS1(2:15) and JS2(2:16) the comparison is carried out with Newton's method and with HM and CM [8]. A mat lab program has been written to implement these methods. We use the following stopping criteria for computer programs:

For every method, we analyze the number of iterations needed to converge to the required solution. The numerical results are reported in the Table 1.

We consider the following problems for a system of nonlinear equations.

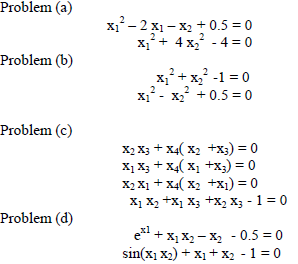CONCLUSIONS

The presented formula (2.15) and (2.16) is simple to understand, easy to program and has the second order of convergence. We contribute to the development of iteration processes and propose of Jarratt’s method. We now obtain a wide general class of Jarratt’s methods which are without memory and have the same scaling factor of function as that Jarratt's method. Numerical tests have been performed, which not only illustrate the method practically but also serve to check the validity of theoretical results we have derived. The performance is compared with Newton method, CM [8] and HM [8].

References

A.M., Ostrowski, (1960). Solutions of Equations and System of Equations, Academic Press, New York.
Ostrowski, A. M., (1966). Solution of equations and system of equations. Academic Press NY, London.
Ortega, J. M. and Rheinboldt, W. C.,(1970). Iterative solution of non-linear equations in several variables, Academic Press, Inc.
Traub, J. F., (1982). Iterative method for the solution of equations, Chelsca Publishing Company, New York.
Dennies Jr, J. E. and Schnabel, R. B., (1983). Numerical methods for uncenstrained optimization and nonlinear equations, Prentice Hall, Englewood Cli_s, NJ.
J.F.Traub,(1964),Iterative Methods for the Solution of Equations, Prentice-Hall, Englewood Cliffs, NJ.
H.T. Kung,J.F. Traub,(1974), Optimal order of one-point and multipoint iteration, Journal of the ACM 21, pp.643-651.
Sharma, R.k. Guha and J. R. , R. (2012), An efficient fourth order weighted-Newton method for systems of nonlinear equations, Springer science +Business Media, LLC.
E.Schroder's, (1870), Uber unendlichviele Algorithm zur Auffosung der Gleichungen, Ann. Math.,2,pp.317-365.
R. Bhel, V. Kanwar and K. Sharma,(2012), optimal equi-scaled families of Jarratt's method, International Journal of Computer Mathematics.