ISSN ONLINE(2319-8753)PRINT(2347-6710)
ISSN ONLINE(2319-8753)PRINT(2347-6710)
| P. Sathishkumar, J. Jancirani, Dennie john and S. manikandan Department of Automobile Engineering, M.I.T, Anna University, Chennai-44, India |
| Related article at Pubmed, Scholar Google |
Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology
This paper is mainly discussing about the mathematical modelling and simulation study of two degree of freedom quarter car model. The state space mathematical model is derived using Newton’s second law of motion and free body diagram concept and the vehicle body along with the wheel system is modelled as a two degree of freedom quarter car model. The performance of the system will be determined by computer simulation using MATLAB/SIMULINK. Passive, semi-active and active suspension systems connected in a single loop and tested under step and single bump input.
Keywords |
| quarter car, state space equation, two degree of freedom, passive suspension, active suspension |
INTRODUCTION |
| The fundamental purpose of ground vehicle suspension system is to maintain continuous contact between the wheels and road surface, and to isolate passengers or cargo from the vibration induced by the road irregularities. These two purposes are responsible for the handling quality and ride comfort, respectively. However, these goals are generally contradictory. It is impossible for passive suspensions to achieve simultaneously a best performance of ride comfort and handling quality under all driving conditions [1]. The vehicle suspension system are categorised into following namely passive, semi active and fully active suspension system. Figure 1.a. shows Conventional suspension systems consist of spring and damper. These parameters spring constant and damping constant are fixed from the design stage itself, so it cannot control. Passive suspension systems with no controllable standard characteristics are the most Widespread on producing vehicles. Firstly, it is caused by an enough simple design, concerning high reliability, absence of necessity of power supply [2]. The problem of passive suspension is if it designs heavily damped or too hard suspension it will transfer a lot of road input or throwing the car on unevenness of the road [3].Many analytical and experimental studies on active and semi-active suspensions have been performed to improve ride quality and handling performance. The results of studies show that active and semi-active suspensions can provide substantial performance improvements over passive suspensions in general [4]. The Electronically controlled active suspension systems can potentially improve the ride comfort as well as the road handling of the vehicle simultaneously [5]. The ride comfort is improved by means of the reduction of the car body acceleration caused by the irregular road disturbances from smooth road [6]. Therefore the active suspension systems are superior in performance than passive and semi-active suspension. |
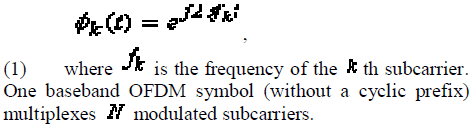
 |
MODELLING OF QUARTER CAR |
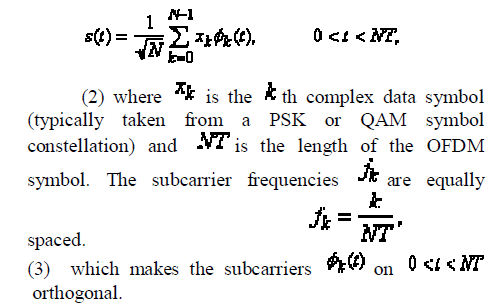
| The vehicle model considered in this study is quarter car model. The quarter car model suspension system consists of one-fourth of the body mass, suspension components and one wheel [7] as shown in Figure 1.The quarter car model for passive suspension system is shown in Figure 1(a). |
| The assumptions of a quarter car modelling are as follows: the tire is modelled as a linear spring without damping, there is no rotational motion in wheel and body, the behaviour of spring and damper are linear, the tire is always in contact with the road surface and effect of friction is neglected so that the residual structural damping is not considered into vehicle modelling [7]. The equations of motion for the sprung and unsprung masses of the passive quarter car model are given by. |
 |
| The equations of motion for the sprung and unsprung masses of the semi active and active suspension quarter car models are identical and given by equation (7) and (8). In case of semi active variable damper will provide a desire force. Other hand the Active suspension system requires an actuatorforce to provide a better ride and handling thanthe passive suspension system. The actuator force, Fa is an additional input to the suspensionsystem model. The model in Simulink was built based on the equations and the actuator and variable damper force iscontrolled by the PID which involves a feedback loop [8]. |
 |
| This equation (11) is the state space equation for active and semi active suspension. |
SIMULATION |
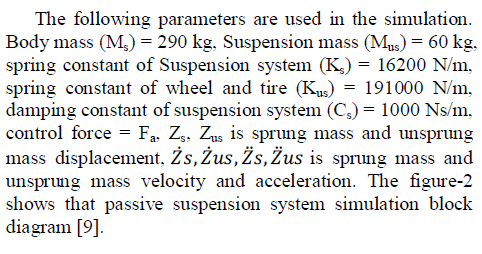 |
 |
| The figure-3 shows that sprung mass displacement with respect to the time trend of passive, semi-active and active suspension system. The peak point of passive is 0.08m and active suspension is 0.05m. and the root mean square value of active is 34% is lower than passive suspension system. The figure-4 shows that unsprung mass displacement with respect to time. |
| The figure-5 shows that suspension deflection or travel graph. This subtraction sprung and unsprung mass displacement. Also known as rattle space. Active suspension deflection 30% is lower than passive suspension |
 |
CONCLUSIONS |
| The passive, semi-active and active suspension system is simulated under Matlab/Simulink environment. From the result of simulation the active suspension is reducing peak amplitude and settling time than semi-active and passive suspension. Also the performance of active suspension is superior to semi-active and which superior to passive suspension. |
ACKNOWLEDGMENT |
| I would like to thank the Anna University and department of Automobile Engineering MIT campus for my research support. Also I thank UGC_RGNF scheme for my funding and research support. |
References |
|