International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Menu

ISSN ONLINE(2319-8753)PRINT(2347-6710)

All submissions of the EM system will be redirected to Online Manuscript Submission System. Authors are requested to submit articles directly to Online Manuscript Submission System of respective journal.

Theoretical and Numerical analysis of immobilised α-chymotrypsin under kinetic control

Vel Alagu1, Alagu Eswari1
  1. Assistant Professor, Department of Mathematics, Sri Ramakrishna Institute of Technology,Pachapalayam, Coimbatore-641010,Tamilnadu, India
Related article at Pubmed, Scholar Google

Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Abstract

In this paper, a mathematical model for immobilized  -chymotrypsin under kinetic control steady-state conditions is discussed. The model is based on diffusion equations containing a non-linear term related to the reaction processes. Analytical expressions for concentrations are derived using Modified Adomian decomposition method. Satisfactory agreement is obtained in the comparison of approximate analytical solution and numerical simulation.

Keywords
Kinetically controlled peptide synthesis, Nucleophile, Internal diffusion and reaction, Non-linear equations, Modified Adomian decomposition method.

I. INTRODUCTION
In recent years, concentration is devoted to enzymes and biocatalysts, particularly monophasic organic solvents [1-5]. Enzymes are normally tightly packed in cellular organelles or in enzyme cascades thus enabling catalytic processes to take place precisely when and where they are needed [6]. Since enzymes are usually insoluble in these systems, unless otherwise conveniently engineered, these are heterogeneous catalytic systems. When comparing the activity of enzymes various organic solvents, it is important to ensure that the roles of external and internal diffusion non-aqueous enzymatic systems do not change for different solvents [7].
These problems may be solved by the use of immobilized enzymes. Immobilization often stabilizes structure of the enzymes, thereby allowing their applications even under harsh environmental conditions [8, 9]. Many predictions of biocatalyst behavior are based on relatively simple physical or chemical interpretations, sometimes combined with knowledge from biocatalysts in aqueous systems. Thermodynamic approaches that consider the distribution of components among the various phases, and their solvation in the bulk (i.e. organic) phase have proved to be particularly useful [10, 11]. A number of applications for enzymes in organic solvents have been developed in chemical processing (particularly for the synthesis of optically active intermediates), food-related conversions and analyses [12].
The study of simultaneous diffusion and reaction is important in order to optimize the catalytic system, which is confirmed by the large number of publications dealing with description and mathematical modeling of this phenomenon [13–20]. Built a model to describe the action of immobilized a-chymotrypsin synthesizing di- or tripeptides in acetonitrile medium under kinetic control [21].
To the best of our knowledge, there are no analytical solutions reported for the molar concentrations of acyl donor and nucleophile. In this paper, we have derived the new analytical expression of concentration of acyl donor and nucleophile. Also we have provided the simple expression of rate consumption for each of the substrates. In addition our analytical results of the molar concentrations are compared with the numerical simulations using Matlab program.

II. MATHEMATICAL MODELING
In acetonitrile medium under kinetic control, the action of  -chymotrypsin synthesizing di- or tripeptides can be expressed by the following enzyme-catalyzed reactions [21]:
Formation of product:
image
image

III. ANALYTICAL DETERMINATION OF THE CONCENTRATIONS OF OF THE ACYL DONOR AND NUCLEOPHILE UNDER STEADY-STATE
The Adomian decomposition method (ADM) is a creative and effective method for exactly solving the differential equations of various kinds. It is important to note that a large amount of research work has been devoted to the application of the ADM to a wide class of linear and nonlinear, ordinary or partial differential equations. The ADM decomposes a solution into an infinite series which converges rapidly to the exact solution. The convergence of the ADM has been investigated by a number of authors [22-27]. In order to solve the boundary value problem, Eq. 9–12, we used the Adomian decomposition method.
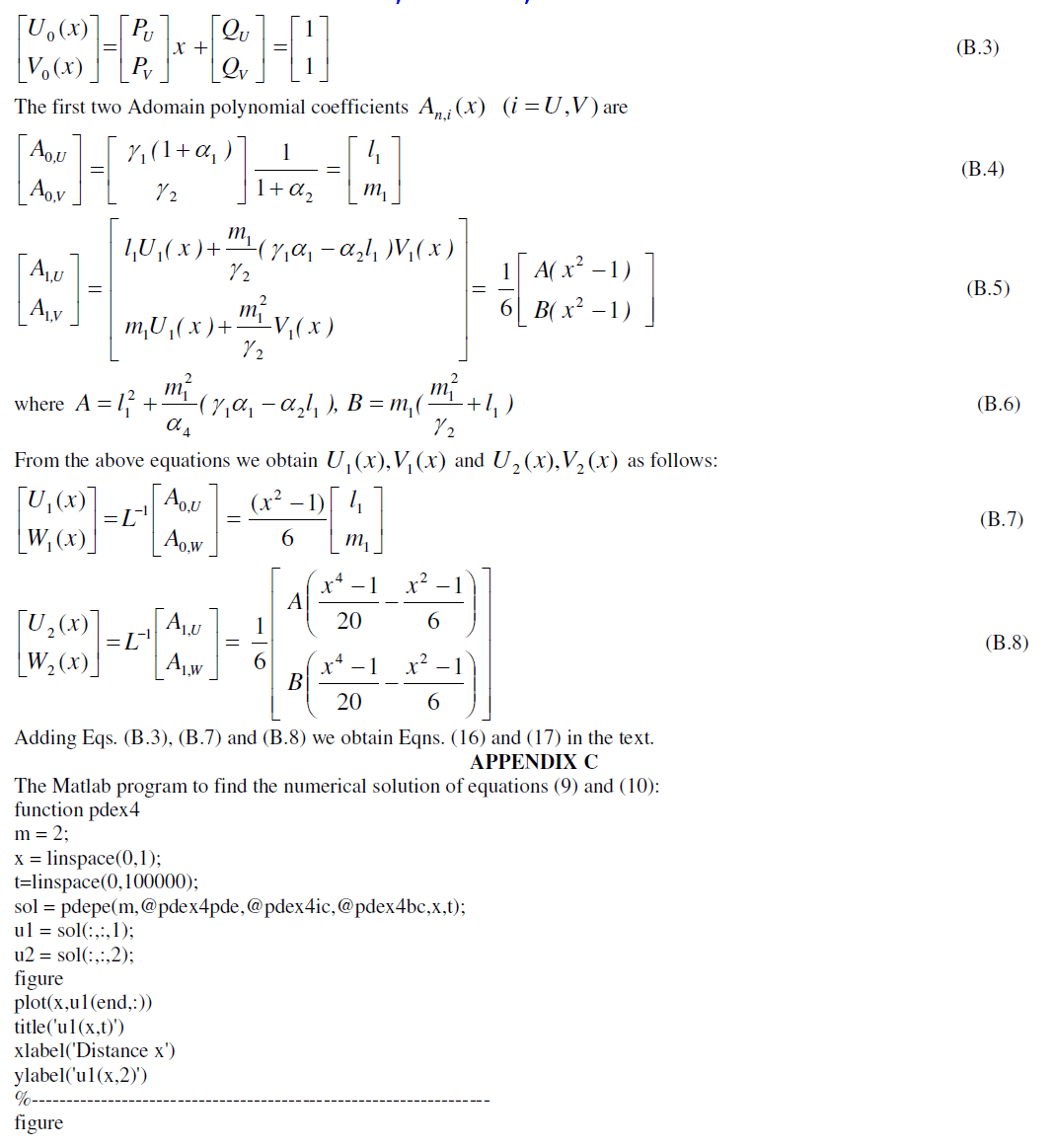
The basic principle of this method is described in Appendix A. Detailed derivations of the dimensionless concentrations U and V of the acyl donor and nucleophiles are described in Appendix B. As a result, we have obtained
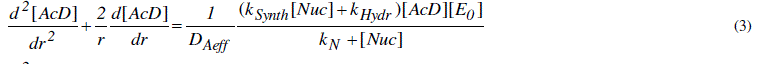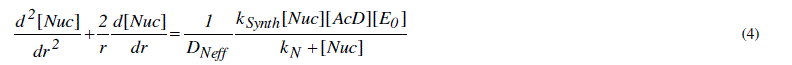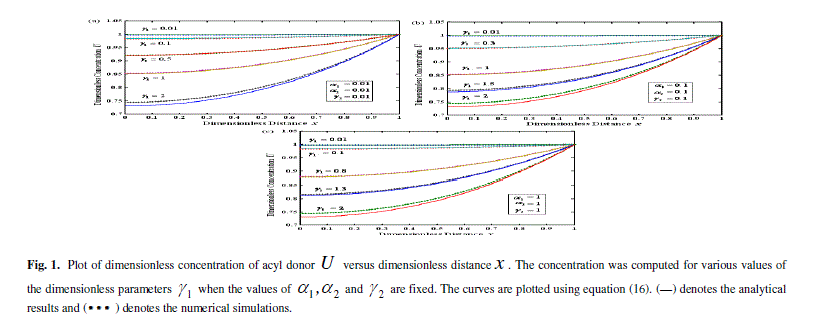
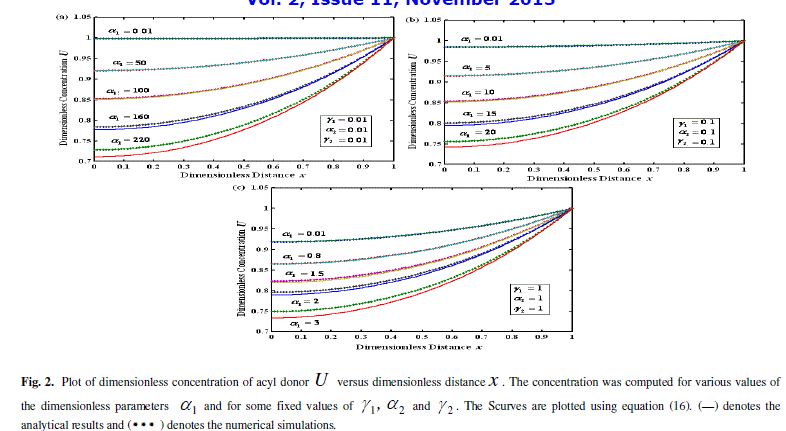
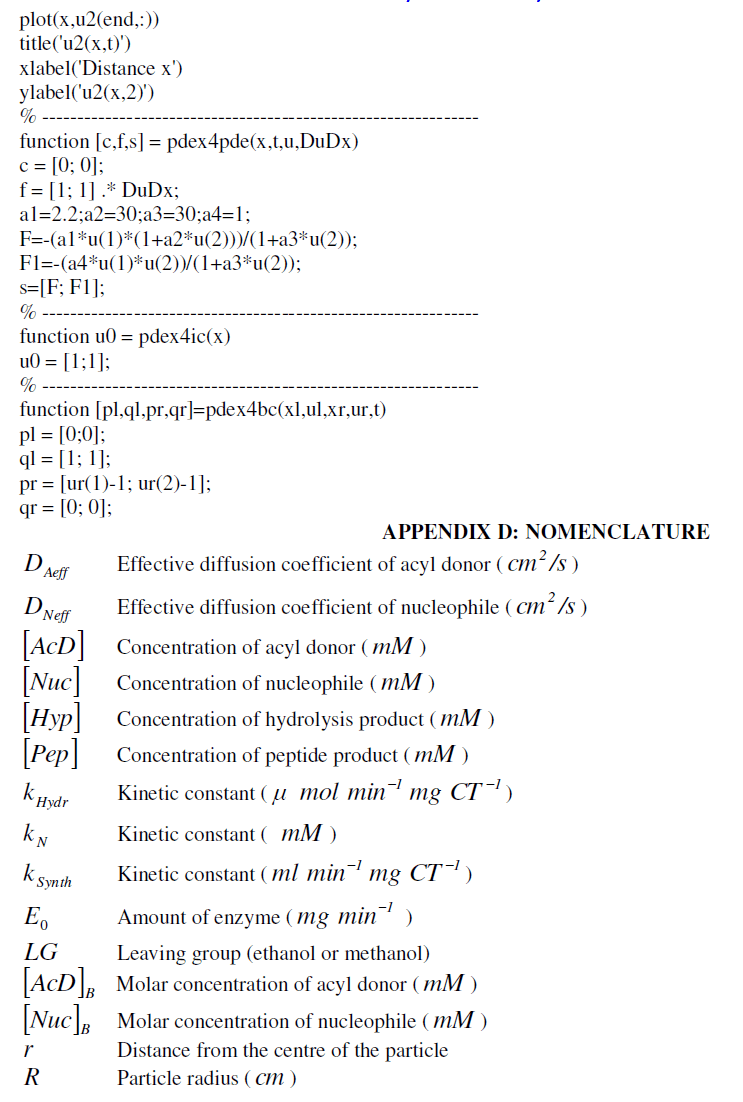
image
image

IV. SIMULATION
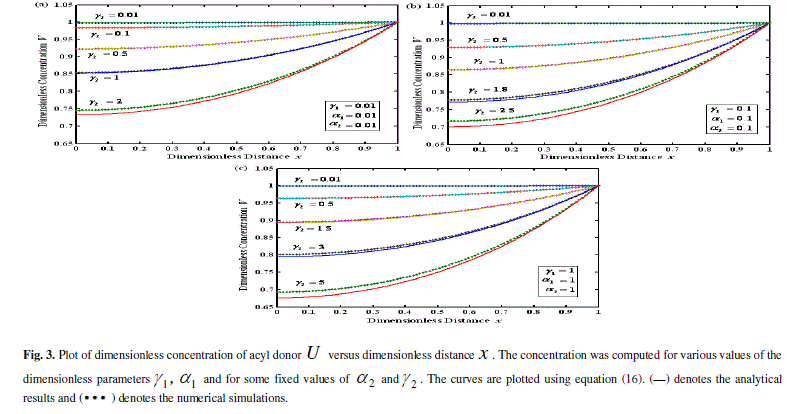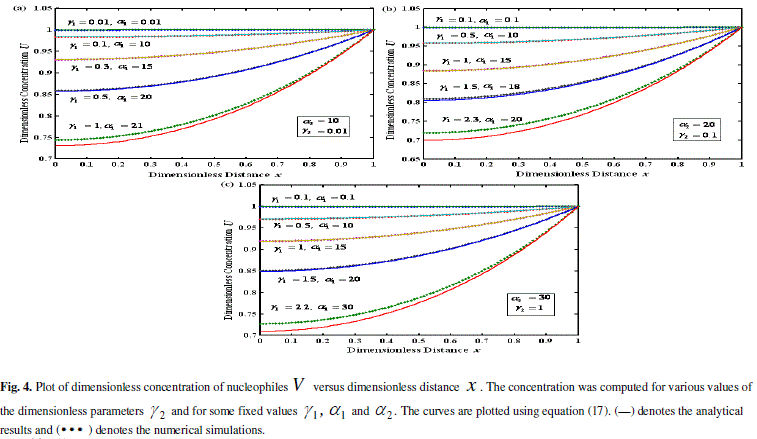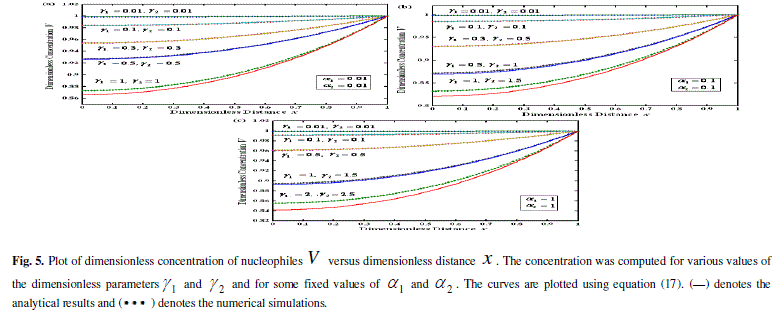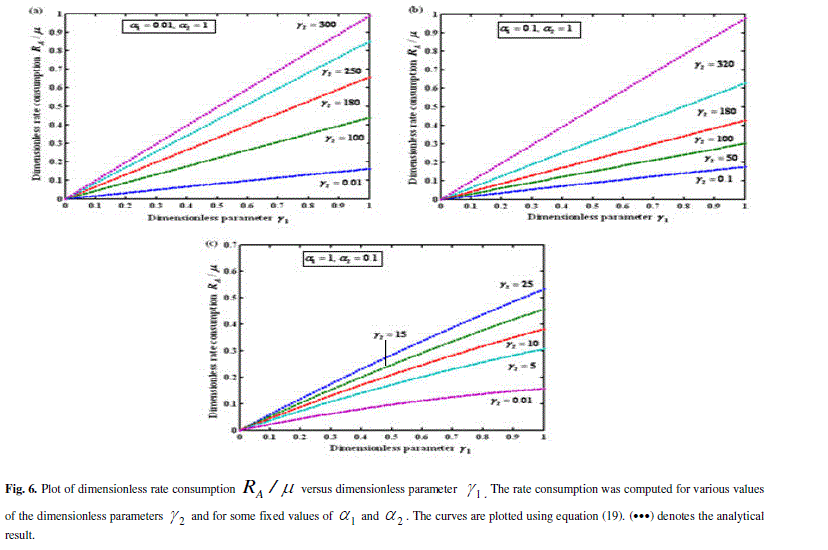image
image
image
image
image
image
image

VI. CONCLUSIONS
In this work, we obtained analytical expressions of concentration of acyl donor and nucleophile for all possible values of dimensionless parameters. The closed analytical expressions of concentrations are obtained using modified Adomian decomposition method. Furthermore, on the basis of the outcome of this work, it is possible to calculate the approximate amounts of rate consumption used for immobilized a-chymotrypsin catalyzed peptide synthesis in acetonitrile medium for all possible values of the parameters. This method is an extremely simple method and it is also a promising method to solve other non-linear equations. The information gained from this theoretical model can be useful for the kinetic analysis of the experimental results and the product distribution.

ACKNOWLEDGEMENTS
The author A. Eswari is very thankful to the Management, Dr. R. Joseph Xavier, the Principal, and Prof. K. Kanagasabapathy, the HOD, Science and Humanities (Mathematics), Sri Ramakrishna Institute of Technology, Coimbatore‐641010, Tamil Nadu, India for their encouragement.
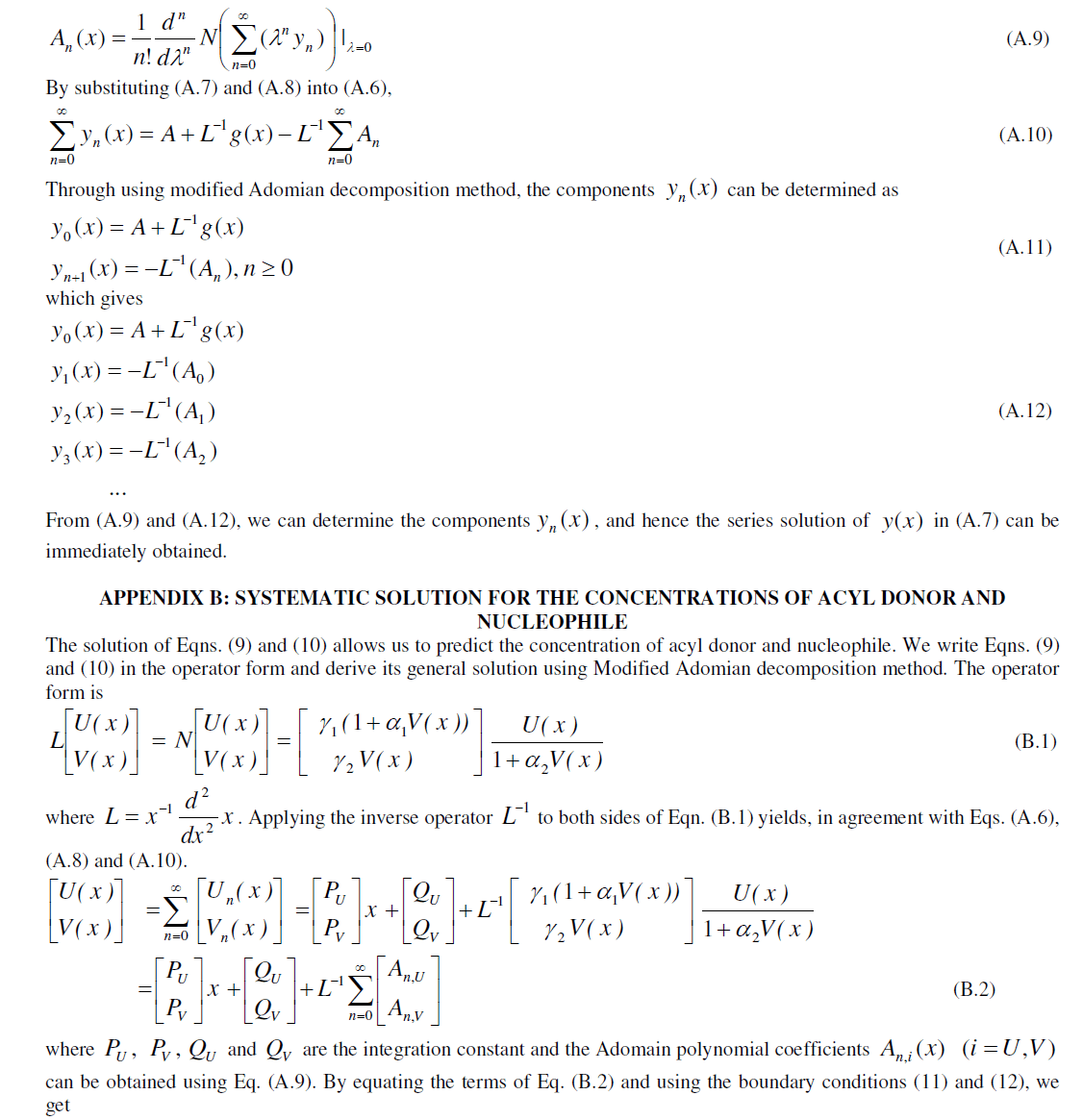image
image
image
image

References
  1. V.M. Paradkar, J.S. Dordick, Aqueous like activity of α-chymotrypsin dissolved in nearly anhydrous organic solvent, J. Am. Chem. Soc. 116, 5009 (1994).
  2. G. Bell, P. J. Halling, B. D. Moore, J. Partridge, D.G. Rees, Biocatalyst behaviour in low-water Systems, Trends Biotechnol. 13, 468 (1995).
  3. P. Adlercreutz, in: A. M. P. Koskinen, A. M. Klibanov (Eds.), Enzymatic Reactions in Organic Media, Chapman & Hall, Glasgow, UK, 1996, p. 9, Chap. 2.
  4. Z. Yang, A. J. Russell, in: A. M. P. Koskinen, A. M. Klibanov (Eds.), Enzymatic Reactions in Organic Media, Chapman & Hall, Glasgow, UK, 1996, p. 43, Chap. 3.
  5. A. M. Klibanov, Why are enzymes less active in organic solvents than in water? Trends Biotechnol. 15, 97 (1997).
  6. R.H. Wijffels, R.M. Buitelaar, C. Bucke, J. Tramper, Working Party on Immobilized Biocatalysts, Guidelines for the characterization of immobilized Biocatalysts, Enzyme Microb. Technol. 5, 304-307 (1983).
  7. S. Kamat, E. J. Beckman, A. J. Russell, Role of diffusion in nonaqueous enzymology. I. Theory, Enzyme Microb.Technol. 14, 265 (1992).
  8. J. Cabral, L. Boross, Tramper J. Applied biocatalysis. Chur: Harwood Academic Publishers, (eds) 1994.
  9. S. D’Souza Immobilized enzymes in bioprocess, Curr. Sci. 77, 69-77 (1999).
  10. E. N. Vulfson, Enzymatic synthesis of food ingredients in low-water media, Trends Food Sci. Technol. 4, 209- 215 (1993).
  11. J. Tramper, M. H. Vemme, H. H. Beeftink and U. von Stockar, eds (1992) Biocatalysis in Non- Conventional Media, Elsevier.
  12. A. M. P. Koskinen and A. M. Klibanov, eds., Enzymatic Reactions in, Organic Media, Blackie Academic & Professional, (1996).
  13. D. Vasic-Racki, M. Gjumbir, Intraparticle mass-transfer resistance and apparent time stability of immobilized yeast alcohol dehydrogenase, Bioproc. Eng. 2, 59 (1987).
  14. A.V. Gusakov, A Fortran Program for Evaluation of the Effectiveness Factor of Biocatalysts in the Presence of External and Internal Diffusional Limitations, Biocatal. Biotransform. 1, 301 (1988).
  15. S. Guzy, G.M. Saidel, N. Lotan, Packed-bed immobilized enzyme reactors for complex process, Bioproc. Eng. 4 239 (1989).
  16. V. K. Jayaraman, A simple method of solution for a class of bioreaction-diffusion problems, Biotechnol. Lett. 13, 455 (1991).
  17. S. Zvirblis, E. Dagys, A. Pauliukonis, Intraparticle Diffusion Describing Factor, Biocatal. Biotransform. 6, 247 (1992).
  18. P. Bernard, D. Barth, Internal Mass Transfer Limitation During Enzymatic Esterification in Supercritical Carbon Dioxide and Hexane, Biocatal. Biotransform. 12, 299 (1995).
  19. S. Sato, T. Murakata, T. Suzuki, M. Chiba, Y. Goto, Esterification activity in organic medium of lipase immobilized on silicas with differently controlled pore size distribution, J. Chem. Eng. Jpn. 30, 654 (1997).
  20. B. A. Bedell, V. V. Mozhaev, D. S. Clark, J. S. Dordick, Testing for diffusion limitations in salt- activated enzyme catalysts operating in organic solvents, Biotechnol. Bioeng. 58, 654 (1998).
  21. Raul J. Barros, Ernst Wehtje, Patrick Adlercreutz, Modeling the performance of immobilized α- chymotrypsin catalyzed peptide synthesis in acetonitrile medium, J. molecular Catalytic B: Enzymatic. 11, 841-850 (2001).
  22. G . Adomian, Convergent series solution of nonlinear equations, J. Comp. Appl. Math. 11, 225–230 (1984).
  23. G. Adomian, Solving the mathematical models of neurosciences and medicine, Math. Comp. Simul. 40, 107– 114 (1995).
  24. G. Adomian, M. Witten, Computation of solutions to the generalized Michaelis-Menten equation, Appl. Math. Lett. 7, 45–48 (1994).
  25. M. Danish, S. Kumar, Approximate explicit analytical expressions of friction factor for flow of Bingham fluids in smooth pipes using Adomian decomposition method, Commun. Nonlinear. Sci. Numer. Simul. 16, 239–251 (2011).
  26. G. Hariharan, K. Kannan, A comparison of Haar wavelet and Adomian decomposition method for solving onedimensional reaction–diffusion equations, Int. J. Appl. Math. Comput. 2, 50–61 (2010).
  27. AM. Wazwaz, The decomposition method applied to systems of partial differential equations and to the reaction–diffusion brusselator model, Appl. Math. Comput. 110, 251–264 (2000).