ISSN ONLINE(2319-8753)PRINT(2347-6710)
ISSN ONLINE(2319-8753)PRINT(2347-6710)
Keyur Thakkar1, Krishna Kumar2, Harshit Trivedi3
|
| Related article at Pubmed, Scholar Google |
Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology
Limitations of air cooling give motivation to apply liquid cooling for high heat flux dissipation because the integrated circuits observe an exponential growth in number of transistors following the conjecture of Moore‟s Law over last few decades. Micro/Mini channel is one of the candidates for high heat flux dissipation. To understand performance of cooling at micro/mini scale, fluid flow & heat transfer need to study. The parameters which affect the behaviour of fluid flow are mass flow rate, specific heat of fluid, geometry & Surface texture of channel would play major role over here. According to scaling law, as compactness increases, heat transfer increases & pressure drop decreases. Increasing the hydraulic diameter from micro to mini scale configurations, we can get heat transfer coefficient sufficient at a relatively lower pressure drop for specific application. It is need to focus on applicability of conventional correlation at this scale, and analyze reasons for deviation from experimental result. So, here single phase liquid flow in mini channel is reviewed.
Keywords |
| Mini channel, Electronic cooling, Single phase laminar flow, Pressure drop, Surface texture |
INTRODUCTION |
| The rapid development in electronic technology and continuous progress leads to compactness in electronic component. First efforts was in early 1950s, the objective of miniaturizing electronics equipment to include complex electronic functions in limited space with minimum weight. Others side of development is an exponential growth of number of transistors in integrated circuits following the MooreâÃâ¬ÃŸs Law over last few decades. Dr.Moore predicted that „The number of transistors on an integrated circuit would double every 18 monthsâÃâ¬ÃŸ, a conjecture referred to as Moore's Law (Moore GE and Fellow L, 1998). Here increases the compactness of electronic components and number of transistor which result in increment the value of internal resistance of component .According to Joule`s law heat dissipation followed the equation I2R . I is current and R is resistance, so it is obvious that as compactness increases internal heat generation increases. So high heat flux dissipation is needed inform of efficient cooling system. As dimensions of electronic components reduce at micro/mini scale, it is necessary for us to use a cooling system which can apply to the same scale. Fluid flow inside micro and mini channel is one of the efficient candidates for small scale cooling. According to [2] Micro and mini channels are classified based on the hydraulic diameter (Dh) as followed. ïÃâ÷ Minichannels: 3 mm ≥ Dh > 200 μm ïÃâ÷ Microchannels: 200 μm ≥ Dh> 10μm Based on scaling law, as compactness increases, heat transfer increases & pressures drop also increases. So, higher heat transfer coefficient comes at the cost of greater pressure drop and hence greater requirement of pumping power. Increasing the hydraulic diameter from micro to various mini scale configurations, we can get heat transfer coefficient sufficient at a relatively lower pressure drop for specific system application. |
| Electronic cooling is possible by various medium like liquid, gas, air. Generally in past year air and water preferred as cooling medium. If we compare both of them, then Air has almost reach its limit about 100 W/cm2 ,because as compare to water, air has less heat caring capacity, also density of air is less than water so with compare to air cooling , water cooling is used for high heat flux removal. Now instead of water many other liquid are used now a days. So here we focus on liquid flow in mini channel. Here, two options are available for liquid cooling ïÃâ÷ Liquid flow with Phase change ïÃâ÷ Liquid flow without Phase change Both the methods have advantages and disadvantages; single phase flow has less pressure drop than two phase flow. Flow boiling of liquid is Single component two phase flow which can give more heat transfer than single phase flow. Here we focus on Single phase liquid flow in mini channel. To understand the heat transfer mechanism, the thermophysical properties of fluid have been comparatively analysed. Fluid flow inside channel represents the nature and behaviour of heat transfer. Convective heat transfer co-efficient is function of fluid property and flow properties. The parameters which affect the behaviour of fluid flow are Mass flow rate, Specific heat of fluid, Geometry & Surface Roughness of channel would play major role over here. |
II. THEORITICAL BACKGROUND OF MINITURIZATION |
| A. Basic Physics of Miniaturization - Scaling Law At micro scale, parameters like surface tension, fluidic resistance and energy dissipation become aggressive and begin to dominate the system. Hence, millimeter and sub-millimeter scale heat dissipation systems require different treatment than conventional macro fluidics. To understand this scaling law, the fully developed laminar flow is considered here. Physical aspects of scaling law in fluid flow. For Laminar Flow Hagen-Poiseuille law for pressure drop is given by |
 |
| B. Mini Channel Heat Exchanger Mini Channel Heat exchanger is a type of compact heat exchanger. The definition of compact is consciously chosen as implying surface area densities about 700 m2/m3[1]. In simple words, Heat exchanger is a device that is used to transfer thermal energy (enthalpy) between two or more fluids, between a solid surface and a fluid, or between solid particulates and a fluid, at different temperatures and in thermal contact. The hydraulic diameter of channel should remain in between 200μm to 3mm to be in range of mini channel according to [2]. |
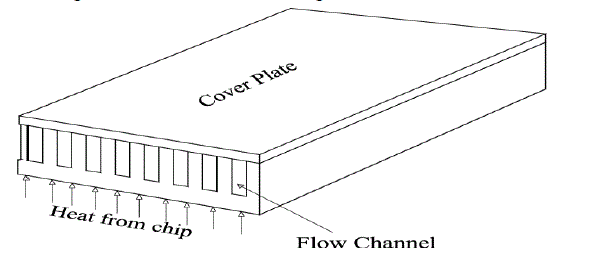 |
| Fig. 1 Rectangular Mini channel |
| Now, with reference to following review from 200μm to 1000μm there is deviation in application of continuum assumption, and small hydraulic diameter can give better heat transfer performance, so it is necessary to understand behavior of fluid flow in this channel range of 200μm to 1000μm. when we go above 1 mm diameter, literature shows good agreement with conventional correlation. |
III. FLUID FLOW & HEAT TRANSFER IN MINI CHANNEL – REVIEW |
| Due to the countless applications of flows in closed conduits such as channels and pipes, a full understanding of developing and developed flows is very important. With respect to thermal aspect heat transfer and pressure drop is key function of channel flow because these define the power input required to drive the flow and the thermal performance of the system. The basic concept of miniaturization was first evolved by D.B.Tuckerman & R.F.W.Pease (1981)[3]. They shows that the heat transfer can be enhanced by reducing the channel height down to micro scale. They were able to reach the highest heat flux of 7.9 MW/m2 with the maximum temperature difference between substrate and inlet water of 71 °C. However, the penalty in pressure drop was also very high, i.e. 200 kPa with plain microchannels and 380 kPa with pin fin enhanced micro channels. As hydraulic diameter of pipe decrease, pumping power increases, due to that pressure drop increases. This is a main disadvantage of miniaturization. R.J Phillips et al. (1987) [4] considered rectangular channels in silicon and used water as coolant. They performed theoretical modeling for fully developed and developing flows and concluded that turbulent flow designs showed equivalent or better performance compared to laminar flow designs. He experimentally studied microchannel heat sinks for laminar and turbulent flows. The heat sink was fabricated using indium phosphide and water was used as the coolant. The channel dimensions were typically w = 220 μm, H = 165 μm and L = 9.7 mm. Thermal resistances of the order of 0.072 Ãâ¹ÃÅ¡C-cm2/W were obtained for very large pressure drops of the order of 2.5 bar. M.M. Rahman and F. Gui (1993) [5] they used rectangular channels etched on silicon and show that for developing flow Nusselt numbers are higher than those predicted from analytical solutions. B.X. Wang and X.F. Peng (1994) [6] conducted forced convection flow and heat transfer experiments in rectangular stainless steel microchannel heat sink. They noticed that the fully developed turbulent regime starts at Re of 1000 to 1500. They also observed that the heat transfer is augmented as liquid temperature was reduced and as liquid velocity Peng & G.P. Peterson (1995, 1996) [7,8] conducted experiments on effect of thermo fluid properties and geometry on convective heat transfer in rectangular stainless steel microchannel heat sink. They found that the transition zone and heat transfer characteristics in laminar and transition flow are influenced by liquid temperature, velocity, Re number and microchannel size. Considered a water flow through rectangular machined steel grooves. The hydraulic diameters investigated ranged between 133 and 367 μm. For Reynolds numbers ranging between 8 and 800, they evidenced higher friction factors with respect to the macro scale predictions; the main result obtained in these works is that the friction factor and the Reynolds number do not seem to be inversely proportional, as indicated by the conventional theory for laminar flow. The authors proposed empirical correlations in order to calculate the friction factor in laminar and in turbulent regime. Y. Zhuang et al. (1997) [9] conducted experiments in impingement on 2-D rectangular microchannels. They suggested new empirical correlation for Nusselt number for the two liquids. M. Mohiuddin and D. Li (1999) [10] show that for lower Re, the required pressure drop is approximately same as predicted by the Poiseuille flow theory. But, as Re increases, there is a significant increase in pressure gradient compared to that predicted by the Poiseuille flow theory. For the fixed flow rate and diameter, Fused Silica microtube requires a higher pressure gradient than a stainless steel microtube, which may be due to either an early transition from laminar flow to turbulent flow or the effects of surface roughness of the microtubes. B. Xu et al.(2000) [11] Compared the experimental data obtained with rectangular microchannels machined in an aluminium plate and bonded with a glass plate with the data obtained by using microchannels etched on a silicon wafer and bonded with a cover. The hydraulic diameters investigated ranged from 46.8 to 344.3μm for aluminium microchannels and from 29.59 to 79.08 μm for silicon microchannels. They concluded that for liquid flow through microchannels with a hydraulic diameter greater than 30 μm the conventional results obtained by using the Navier– Stokes equation for an incompressible, Newtonian fluid in the laminar regime agree very well with the experimental data. F. Debray et al.(2001) [12] Presented measurements of the friction factor in a flat rectangular microchannel having a hydraulic diameter of 590 μm for Reynolds numbers ranging between70 and 6300. Their results confirmed the prediction of the conventional theory. C. Gillot et al.(2002) [13] showed that relation of pressure drop & thermal resistance with flow rate by doing experiment on single phase water cooling in rectangular mini channel having dimension of 2 mm depth, length is 18mm and width is 51 mm. Reynaud et al. (2005) [14] indicated measurements of the friction and heat transfer coefficients in 2D minichannels of 1.12 mm to 300 μm in thickness for Copper channel. Transition to turbulence occurs for Reynolds number between 3000 and 5000. The experimental data exhibit a slight decrease of the Poiseuille number between the case 1.12 mm and 540 μm and decreases between 540 μm and 300 μm. The friction factor is estimated from the measured pressure drop along the whole channel. The experimental results are in good agreement with classical correlations relative to channels of conventional size. The observed deviations are explained either by macroscopic effects (mainly entrance and viscous dissipation effects) or by imperfections of the experimental apparatus. C.Y.Yang , T.Y.Lin (2007) [15] for Dh 123-962 μm, Channel material Stainless steel material show that the conventional heat transfer correlations for laminar and turbulent flow can be well applied for predicting the fully developed heat transfer performance in microtubes. Developing Nusselt numbers for 962 μm tube agree well with those predicted by the Shah and Bhatti [R.K. Shah, M.S. Bhatti, Laminar convective heat transfer in ducts]. W. Qu, & I. Mudawar(2007) [16] measured the pressure drop of water flowing in a single-phase microchannel heat sink. They found that the pressure drop was in good agreement with the predicted values of the conventional theory for continuum flows. The change in the slope of the pressure drop with Reynolds number was attributed to the temperature dependence of water viscosity. |
| X.L Xie et al. (2007, 2009) [17, 18] show numerical study of minichannel heat sink with bottom size of 20 mm × 20 mm is analyzed numerically for the single-phase laminar flow of water as coolant through small hydraulic diameters and a constant heat flux boundary condition is assumed and validate result with Conventional correlation and same procedure was repeated for turbulent flow for single phase water flow. They also study about effect of dimension of channel in heat transfer, pressure drop in both case laminar and turbulent flow. J. Wilk Joanna(2009) [19] did an experimental investigations of convective mass/heat transfer in short circular channel with small inner diameter 1.5 mm Nickel Channel at Re numbers ranging from 20 to 250 were performed. Mean values of convective mass transfer coefficients were measured using electrochemical technique – limiting current method. The results of dimensionless mass transfer coefficient Sh were compared with conventional theory. It was observed the increase of experimental Sh in compare with data from classical correlation relative to channels of conventional size. The observed deviations could be explained by the entry effects. It is difficult to say if the channel size effect on the mass transport. Z. Zhou et al.(2010) [20] show for transient heat transfer under high heat flux density which varies from 150 W/cm2 to 200 W/cm2. Experimentally they show that for Dh 1.5mm in Aluminium rectangular channel, The surface temperature is a function of thermal resistance and thermal capacity of the heat sink. The temperature of the cooling water at outlet also rises quickly at the startup period of heating, and then becomes stable. The temperature increases are related to the heat flux density and the rate of the cooling water. The pressure drop across the heat sink decreases slightly with time at high heat flux since the water viscosity reduces with the increasing temperature T.D Teng et al. (2011) [21] showed the heat transfer rate obtained from micro channel heat exchanger is higher than mini channel heat exchanger, but pressure drop obtained in micro channel heat exchanger is higher than mini channel heat exchanger. (Because pressure is inversely proportional to cross sectional area on which force is exerted). Effectiveness obtained from micro channel heat exchanger was 1.3-1.53 times of that obtained from mini channel heat exchanger. This is because as per effectiveness, it is ratio of actual heat transfer to the maximum possible heat transfer & actual heat transfer is more in case of micro channel because of comparatively high Nu number in micro channel. |
 |
| So, , , (4) So it indicate that the as hydraulic diameter decrease the convective heat transfer increase. They also verified the fact on micro level that pressure drop obtain from the hot side is always higher than that obtained from cold side, it indicate that mass flow rate of cold side is less than that of hot side. It is indicated that the pressure drop is also function of inlet temperature of hot side, as it increases, the pressure drop decreases. Moharana et al. (2011) [22] with Copper material and Dh is 0.907mm show that Simultaneously developing flows provide very high heat transfer coefficients in the entrance regions and therefore of interest for mini/micro scale for high heat flux removal application. Flow Re was varied from150 to 2500 while inlet flow Prandtl number was maintained in the range of 3-4. The square root of cross-section area of the fluid flow was also explored as a possible length for scaling; no significant improvement was observed by this choice of the length scale, in the range of the experimental conditions at this scale. The variation of local Nusselt number along the axial direction also gets affected due to the parameter M which is heat conduction number. B. Daia et al.(2014) [23] used ethanol as fluid and rectangular channel 0.715 mm and for circular channel 0.86 mm was hydraulic diameter. Multi port tubes were used & results showed that the Nusselt numbers decrease with the increase of inlet temperature and heat flux. Furthermore, the scaling effects of conjugate heat transfer; entrance effects and temperature dependent viscosity variation on the convective heat transfer are significant. Based on the experimental data, new correlations for the Nusselt numbers were obtained considering the scaling effects for the multiport tubes. |
IV. IMPACT OF SURFACE TEXTURE ON FLUID FLOW BEHAVIOR |
| Surface Texture is the local deviations of a surface from a perfectly flat plane. The measure of the surface texture is generally determined in terms of its roughness, waviness and form. Surface texture is one of the important factors that control friction and transfer layer formation during fluid flow. Surface Textures can be isotropic or anisotropic. Here we observe the behavior of heat transfer and pressure drop in single phase liquid flow which is influenced by surface roughness. |
| S.G Kandlikar ,S. Joshi & S. Tian (2003) [24] studied the effect of roughness on heat transfer and pressure drop characteristic of stainless steel tube of inner diameter of 0.62mm and 1.067mm. Heat fluxes as high as 116 kW/m2 and 35 kW/m2 are obtained for 0.62 mm and for 1.067 mm tubes respectively. The outlet temperature of water being 36°C for both 0.62 mm and 1.067mm tubes for the lowest mass flow rate. In this experiment, the flow is hydro- dynamically fully developed while it is in the thermally developing region. The velocity and thermal entry lengths are calculated. The fully developed hydrodynamic entry length is 57 mm and the thermal development length is 380 mm for 0.62 mm and 115 and 760 mm for 1.067 mm tube at 2300 Reynolds number. Hence it could be stated that for small diameter tubes, the effect of surface roughness has a larger impact on the heat transfer characteristics. Zhao and Z. Liu (2006) [26]conducted pressure drop studies on smooth quartz-glass tubes and rough stainless steel tubes of varying diameters. They observed that in the laminar region, experimental results agreed well with theoretical values. However, early transition at Reynolds numbers ranging from 1100 to 1500 (for smooth micro-tubes) was recorded. For rough micro-tubes (with ε/D = 0.08), laminar theory agreed only until the Reynolds number of 800, where similar early transition was observed. Y. Perry & S.G Kandlikar (2008) [27] shows the critical review for validation of experimental result with conventional correlation. They classified the survey based on experimental result compared with classical theory. 1. Under-predicted by classical theory 2. Over-predicted by classical theory 3. Well predicted by classical theory They give the reason for all three cases and source of error as well like, at low Reynolds numbers, the increase in temperature can be very large across a microchannel, causing a variable property effect. The large temperature gradient implies that the thermo-physical properties cannot be assumed as constant. This causes the bulk temperature of the fluid to vary in a non-linear form in the flow direction, which may cause deviations between experimental data and theoretical predictions the possible error that is often not taken into consideration is axial conduction. From all above Survey we come to know the importance of the non dimensional number like Re, Nu, Pr, Po and their physical interpretation. Type of fluid and fluid flow along with length of flow will help us to determine Convective heat transfer coefficient & also effect of inner surface roughness on pressure drop fluid flow and heat transfer. In mini channel range 200μm to 1 mm is very important to understand as per scaling law. Y. Perry, T. Brackbilla, S.G Kandlikar (2010) [25] give Comparison of Roughness Parameters for Various Microchannel Surfaces in Single-Phase Flow. They found, the average roughness Ra parameter is often used in microfluidic applications, but this parameter alone is insufficient for describing surface roughness. So they introduce new roughness parameter εFp. |
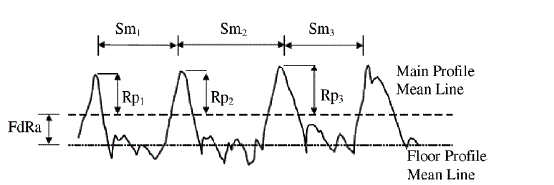 |
| Rsm represents the mean spacing of profile irregularities. This parameter depicts the pitch of the surface profile. Smi (i=1.2.3) represents the peak and valley pairs of the profile, the distance between when the data drops below the dead zone to where the data rise above the dead zone. The maximum profile peak height, Rp represents the distance between the mean line and the highest point of the profile. The maximum profile valley, Rv is a similar parameter and represents the distance between the mean line and the lowest point of the profile. Another useful surface parameter is FdRa, which represents the floor distance to mean line. This roughness parameter is equal to the sum of the roughness parameters RP and FdRa: |
| It is interesting to note that surfaces resulting from different machining processes or etches could have a similar average roughness value but have values that do not match for εFp. The copper ground surface has an average roughness equal to 0.31 μm, and the stainless steel fly cut surface has a similar Ra value of 0.30 μm. However, the εFp value for the copper ground surface is higher than that of the stainless steel. The εFp value for the stainless steel fly cut surface is 1.07 μm, while that of the copper surface ground sample is 1.34 μm. Thus, the εFp parameter would provide a more accurate parameter for modeling the effects of surface roughness on fluid flow. |
V. CONCLUSION |
| In single phase developing laminar flow, reduction in hydrodynamic entrance length gives higher heat transfer as reduction in thermal resistance. Fully developed flow gives more heat transfer than developing flow. , The Nusselt number and heat transfer coefficient increases with increase in mass flow rate. Nu numbers decrease with the increase of inlet temperature and heat flux. , The scaling effects of conjugate heat transfer; entrance effects and temperature dependent viscosity variation on the convective heat transfer are significant. , Relative roughness at micro scale of two channel may have same values even though they made by different machining process. So to differentiate their surface roughness accurately new parameter is proposed Roughness parameter εFp. , Surface roughness can increase the heat transfer and pressure drop, but this effect more accurately as we go towards micro scale. , Some of result of experiment cannot match with conventional theories so, continuum assumption would be one of the responsible factors for that. Here at mini-micro level continuum assumption cannot always be applied. Continuum assumption can be applied at mini level above 1mm as shown in study even though from 200μm to 0.9mm some deviation can be found. So for application of the governing equation, the experimental condition, and dimension of channel, surface roughness and due to it friction fraction all these factors have very high impact on application of continuum assumption. , At micro-mini level, mean free path between molecules reduce and we cannot get point property for particular time period, there is randomness in that value, the reasons for fluctuating of that property is govern by Knudsen number (Kn). It is ratio of the mean free path to the length scale (sample length). So due to miniaturization the distance between those molecules reduce & collision among them increases. So as go towards micro scale there is deviation in Experimental result from conventional co-relation. Results are under predicted, over predicted and well predicted. Under prediction and over prediction occur because of avoiding axial conduction. Continuous changing in Thermo physical properties also responsible for unmatched result. , For Governing of heat transfer co-efficient following parameters are need to be focused. o Mass flow rate o Pumping power o Surface texture o Critical Reynolds Number o Types of fluid flow o Entrance length for developing flow , Turbulent boundary layer develops much faster than laminar boundary layer because of rapid diffusion of momentum and heat due to turbulence. So Turbulent flow gives more heat transfer on penalty of pressure drop than laminar flow. |
| There is future Scope for research in reduction in pressure drop and thermal resistance and increase heat transfer co-efficient by different enhancement techniques at micro/mini scale. |
ACKNOWLEDGEMENT |
| I express my deep sense of gratitude to my guide Prof. Keyur Thakkar & Ass.Prof. Krishna Kumar for helping me throughout. |
References |
|