ISSN ONLINE(2319-8753)PRINT(2347-6710)
ISSN ONLINE(2319-8753)PRINT(2347-6710)
| R. T. Matoog Assistant Professor. Department of Mathematics, Faculty of Applied Sciences,Umm Al-Qura University, Makkah, Saudi Arabia |
| Related article at Pubmed, Scholar Google |
Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology
In this work, the existence of a unique solution of mixed integral equation (MIE) of the second kind is considered, in the space ( ) [0, ] p L C T , where in the domain of integration and t [0,T ] is the time. The kernel of position is considered in a generalized potential form. A numerical method is used to obtain system of Fredholm integral equations (SFIEs). The existence of a unique solution of this system can be proved. Finally, many special cases are considered and established from the work and some numerical results are considered
Keywords |
| Fredholm- Volterra integral equation (F-VIE), generalized potential kernel, degenerate method, linear algebraic system. |
| MSC: 45 B05, 45G 10, 65R |
INTRODUCTION |
| Many problems of mathematical physics, engineering and contact problems in the theory of elasticity, fluid mechanics and quantum mechanics lead to one of the form of the integral equations. In [1], Abdou used the separation of variables method to solve the F-VIE of the first kind in the space |
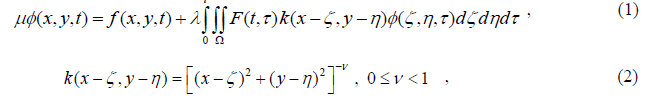 |
| in the space |
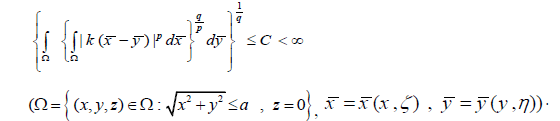 |
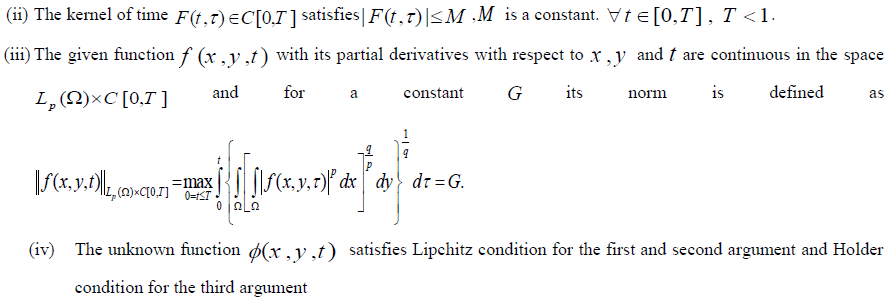 |
| Theorem (1) (without proof): The IE (1) has an existence and unique solution under the condition |
| In this section, we represent the position kernel in the form of a generalized W-SIf. In this aim, after using the polar coordinates in Eqs. (1), (2), we obtain |
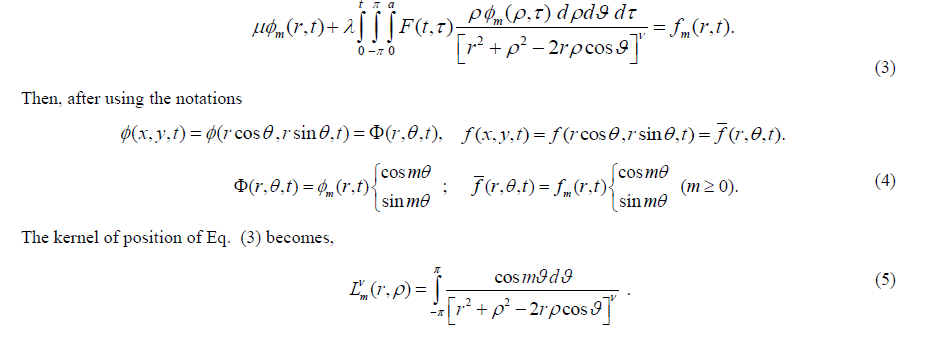 |
| Moreover, using the following three formulas, see Bateman and Ergyli [5, 6], |
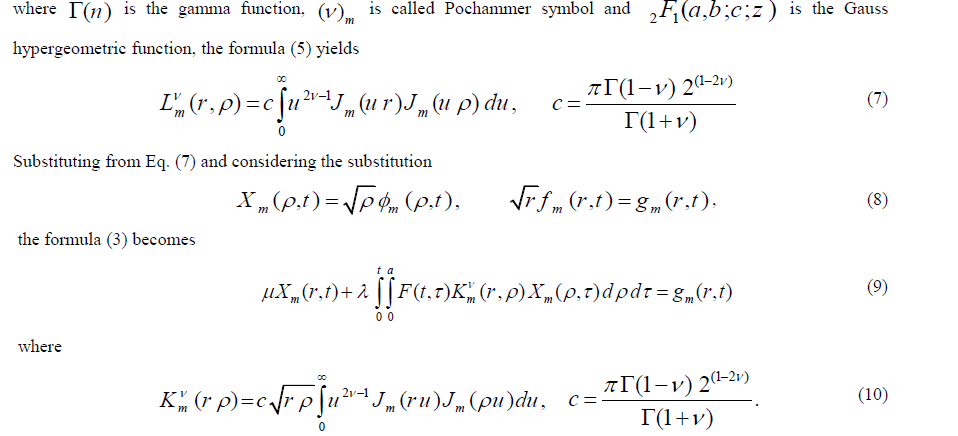 |
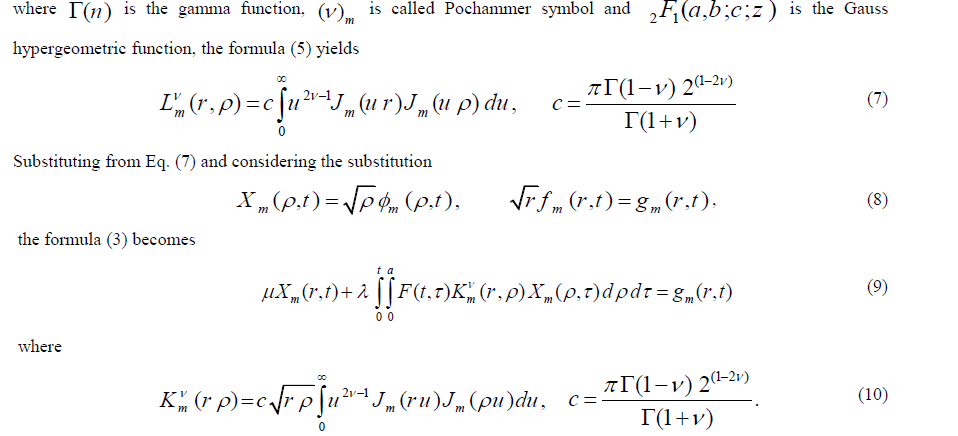 |
| The position kernel (10) takes a general form of W-SIf. |
| 3. On the discussion of the W-SIf: |
| We derive many special and new cases from the W-SIf of (10) |
(1) Logarithmic kernel: Let, in (10) 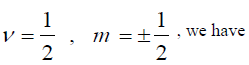 |
 |
 |
 |
 |
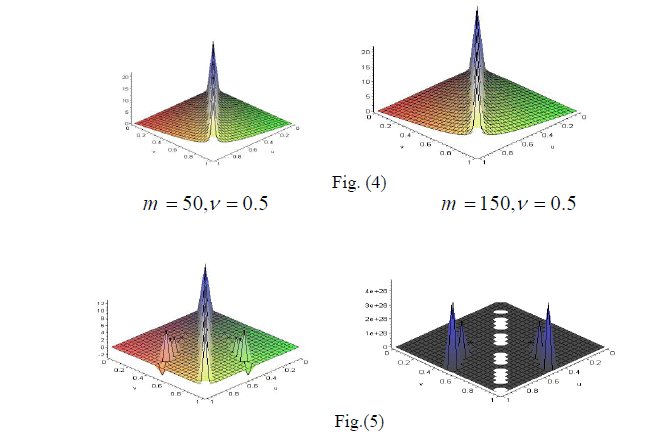
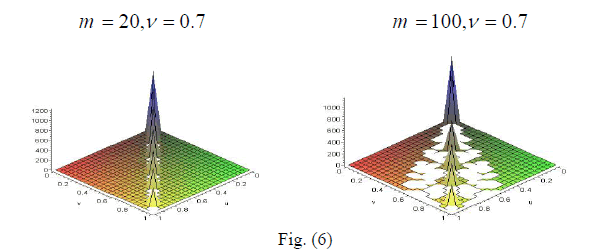
| From the previous figures of Carleman function we deduced that as ï® increases the cracks in the material increase. (3) Elliptic kernel: Let, in (10), |
 |
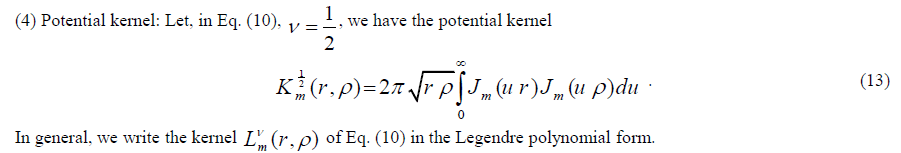 |
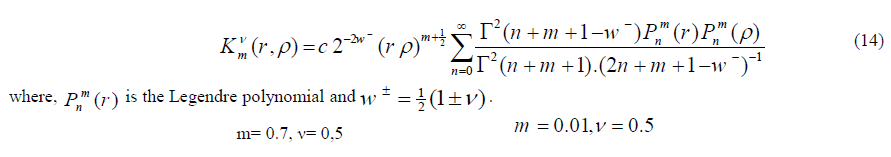 |
 |
| General cases: Here, the W-SIf is representing generalized potential form , and as special cases we consider the following: |
 |
| Theorem (2): The structure of the kernel W –SIf, represents Cauchy problem for the first order and nonhomogeneous wave equation for the second order. Proof: To prove this we differentiate Eq. (12) with respect to r andï² respectively, and then adding the result to get |
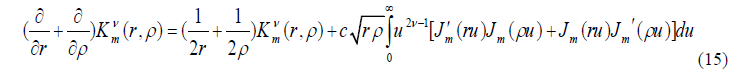 |
| Using the two famous relations, see Bateman and Ergelyi [6] |
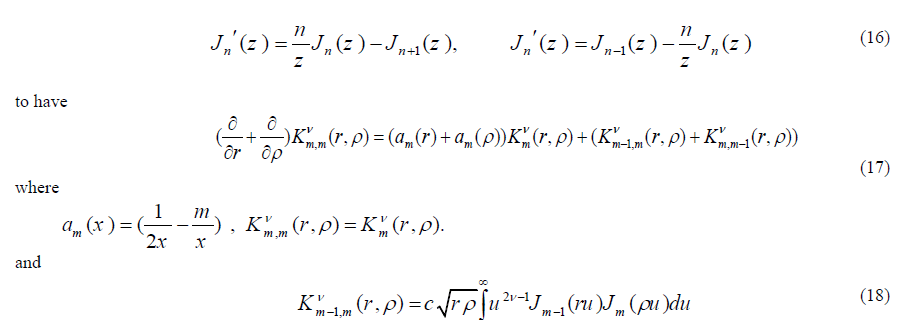 |
 |
| The above formula represents a nonhomogeneous wave equation. So, the second derivative of the generalized potential kernel represents a nonhomogeneous wave equation when m≠0.5. |