ISSN ONLINE(2319-8753)PRINT(2347-6710)
ISSN ONLINE(2319-8753)PRINT(2347-6710)
| Jyoti Prasad Singha Thakur1, Amit Mukhopadhyay2 Asst. Prof., AEIE, Bankura Unnayani Institute of Engineering, Bankura, West Bengal, India1 Asst. Prof., ECE, Bankura Unnayani Institute of Engineering, Bankura, West Bengal, India2 |
| Related article at Pubmed, Scholar Google |
Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology
The present work is an attempt towards achieving an alternative design algorithm for a two-loop autopilot which would involve simpler and less complicated relations, requiring lesser computational efforts and to some extent be flexible in achieving different deflection, deflection rate and body rate demands in specific flight conditions. The design approach in [2] is based on a two-loop lateral autopilot configuration in pitch plane developed as an equivalent flight path rate demand autopilot system. The key idea of this design approach is to achieve a preliminary set of control gains (Kp, Kq) on the basis of a simplified lateral autopilot in yaw plane using an ideal actuator system. This developed approach has been verified by evaluating the performance of the designed autopilots at a typical operating point. In this approach simulation of body rate, fin deflection and fin deflection rate has been observed. An attempt has been made to determine the effect of the different values of control gains on such body rate, fin deflection and fin deflection rate. In this approach sensitivity of the control gains have been studied. It has been studied how the change in control gains affect the frequency domain performance.
Keywords |
||||||||||||
| Autopilot, Control Gain, Critical Gain Margin, Critical Phase Margin. | ||||||||||||
INTRODUCTION |
||||||||||||
| Autopilot is an automatic control mechanism for keeping the spacecraft in desired flight path. An autopilot in a missile is a close loop system and it is a minor loop inside the main guidance loop. If the missile carries accelerometer and rate gyros to provide additional feedback into the missile servos to modify the missile motion then the missile control system is usually called an autopilot. When the autopilot controls the motion in the pitch and the yaw plane, they are called lateral autopilot. For a symmetrical cruciform missile pitch and the yaw autopilots are identical. The guidance system detects whether the missile is flying too high or too low, or too much to the left or right. It measures the deviation or errors and sends signals to the control system to minimize the errors. The lateral autopilot of a guided missile is a servo system delivering lateral acceleration (latax) according to the demand from the guidance computer. For aerodynamically controlled skid to run missile the autopilot activates to move the control surfaces suitably for orienting the missile body with respect to the flight path. This action generates angle of attack and consequently latax for steering the missile in the desired path[1].Missile autopilot design techniques have been dominated by classical control methods over the past several decades and a number of autopilots with two-loop and three-loop control configuration have been designed with their own merits and limitations. G.Das et al has suggested distinct approaches of designing two loop lateral autopilot[2,3].In [2] controller parameters are obtained quickly from a set of parametric equations involving performance measures, the aerodynamic characteristics of the plant and the available actuator. In [2] two design situations have been considered .The first design situation is the selection of actuator bandwidth for a given performance specifications of the controlled missile and in the second design, salient airframe parameters are derived for the given closed loop specifications. In [2] G.Das et al has suggested a systematic design methodology to design the control gains of two-loop lateral autopilot based on frequency domain performance specifications. Expressions are derived for GM, PM, GCF and PCF in parametric form are involving both aerodynamic and other sub-system parameters. For any operating point on the missile flight path envelope, the two desired control gain can be achieved using a developed algorithm by an iterative approach. The resulting design achieves the desired stability margins demanding specific fin deflections, fin rate and body rate demands. The design algorithm however, does not provide any explicit means to achieve some control over control surface deflections, their rates and the missile body rate which might be important in realistic flight conditions to meet the available system hardware constraints of to make a better or the best of the hardware resources implemented in the flight control system. The present work is a modest attempt towards achieving an alternative design algorithm for a two-loop autopilot which would involve simpler and less complicated relations, requiring lesser computational efforts and to some extent be flexible in achieving different deflection, deflection rate and body rate demands in specific flight conditions. The design approach in [2] is based on a two-loop lateral autopilot configuration in pitch plane developed as an equivalent flight path rate demand autopilot system. The aerodynamic transfer functions used are in parametric form and all force and moment coefficients etc are in the semi non-dimensional form in the pitch plane. For the purpose of design and analysis, the present work uses an equivalent two-loop flight path rate demand autopilot system in the yaw plane. This model has been derived using the equations of motion for a missile as presented in[1] and with same control surface conventions and using all aerodynamic derivatives in parametric form in the yaw plane. Design situations considered is similar to that in [2] for a specified actuator system (given ωa, ζa ). The key idea of this design approach is to achieve a preliminary set of control gains (Kp, Kq) on the basis of a simplified lateral autopilot in yaw plane using an ideal actuator system with the help of much simplified relations developed in parametric form involving aerodynamic parameters only and necessitating less computational effort in evaluating the stability margin values. Accordingly, parametric expressions have been derived for GM, PM, GCF and PCF of autopilot with ideal actuator. Again parametric expressions have been derived for GM, PM, GCF and PCF of autopilot with actuator, having same control gains (Kp, Kq). There will be a loss of GM and PM. This loss of GM and PM should be compensated by varying the control gains (Kp, Kq) so as to meet the desired performance specifications. This developed approach has been verified by evaluating the performance of the designed autopilots at a typical operating point for four different types of missile with aerodynamic data. In all four cases value of Kp is obtained from parametric equations where as Kq is chosen in iterative process so as to designed autopilot meet the desired performance specifications. In this approach simulation of body rate, fin deflection and fin deflection rate has been observed. An attempt has been made to determine the effect of the different values of control gains on such body rate, fin deflection and fin deflection rate. In this approach sensitivity of the control gains have been studied. It has been studied how the change in control gains affect the frequency domain performance. | ||||||||||||
II. MISSILE AUTOPILOT OVERVIEW |
||||||||||||
| Guided missiles have assumed much importance in recent years. A guided missile is one which receives steering commands from the guided system to improve its accuracy. Guided action for guided missiles may be defined as the process of gathering information concerning the flight of a missile towards a given objective or target and utilising this information to develop manoeuvring commands to the control system of the missile. Guidance system functions by comparing the actual path of the missile with the desired path and providing commands to the control system which will result in manoeuvring the missile to its desired path. Guidance system actually gives command to the autopilot to activate the controls to achieve the correction necessary. Autopilots are closed loop system and these are minor loops inside the main guidance loop. An autopilot may be defined as the missile control system which modify the missile motion according to accelerometer and / or gyros feedback which provide information about the missile acceleration and body rate respectively. A lateral autopilot receives guidance command from the guided system of the missile to produce desired missile acceleration in lateral planes to follow the guidance path needed to the target. The autopilot responses to guidance system demand by deflecting the control surfaces of the missile for aerodynamic controlled missiles. The deflections in control surfaces produce change in missile angle of incidence. If the incidence angle is changed, the forces acting on the missile body changes and it results in change in missile acceleration. The two loop autopilot system uses two loops to feedback information of missile motion to the forward path of the autopilot. One loop is involved with body rate information which is fed back using one rate gyro. The other is the missile acceleration, sensed using accelerometer and provides the main feedback. The autopilot system results in change in missile motion. So, modelling of missile airframe dynamics is an important part of configuring an autopilot system. Missile dynamics is of non linear type. For configuring missile dynamics in transfer function form the missile airframes are trimmed and then linearized. | ||||||||||||
III. PROCESS MODEL |
||||||||||||
| The reference axis system standardized for Guided Missile Industry is centered at the Centre of Gravity (c.g.) of the missile and is fixed in the body as shown in Fig 1. The X-axis is called the “Roll Axis”, which defined as positive in forward direction. The Y-axis is called the “Pitch Axis”, which is defined positive outwards and to the right of the Roll Axis, when viewed from the rear end of the missile.The Z-axis is called the “Yaw Axis”, which is defined positive downwards in the plane of symmetry & forms a right handed orthogonal system with the other two axes. | ||||||||||||
| Block diagram of two-loop lateral autopilot in yaw plane will be as follows | ||||||||||||
IV. MATHEMATICAL MODEL OF TWO-LOOP AUTOPILOT IN YAW PLAN |
||||||||||||
| The open loop transfer function of the autopilot for loop opened at X1 is given by, | ||||||||||||
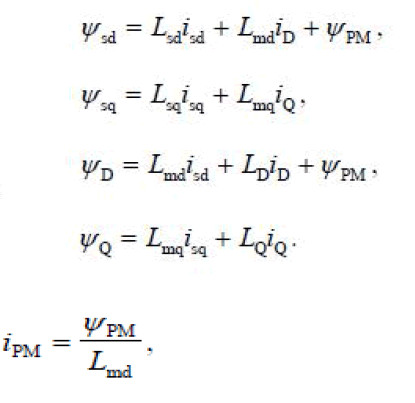 |
||||||||||||
| Magnitude of GX1 (jw) is | ||||||||||||
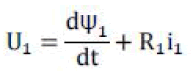 |
||||||||||||
| Phase angle of GX1 (jw) is | ||||||||||||
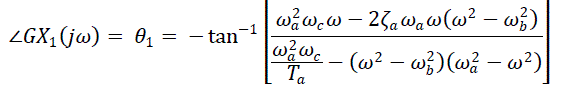 |
||||||||||||
| Condition for Phase crossover frequency (ω=ωpx1): = | ||||||||||||
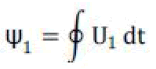 |
||||||||||||
 |
||||||||||||
| Condition for Gain crossover frequency (ω=ωgx1): = | ||||||||||||
 |
||||||||||||
| Phase Margin: | ||||||||||||
 |
||||||||||||
| Autopilot Loop Opened at X2 : | ||||||||||||
| The open loop transfer function of the autopilot for loop opened at X2 is given by, | ||||||||||||
| Magnitude of GX2 (jw) | ||||||||||||
 |
||||||||||||
| Phase angle of GX2 (jw) | ||||||||||||
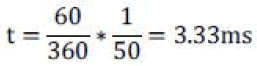 |
||||||||||||
| Condition for Phase Crossover Frequency (ω=ωpx2): | ||||||||||||
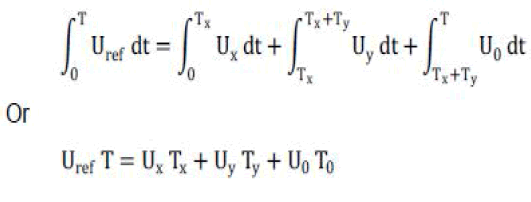 |
||||||||||||
| Gain Margin : | ||||||||||||
 |
||||||||||||
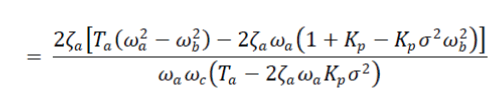 |
||||||||||||
| Condition for gain crossover frequency (ω=ωgx2) | ||||||||||||
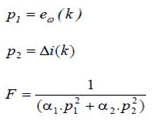 |
||||||||||||
| Phase Margin : | ||||||||||||
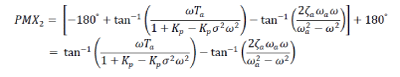 |
||||||||||||
| Design Objective: To obtain the control gains Kp and Kq for maximum possible gain cross over frequency (GCF) satisfying desired performance specifications. | ||||||||||||
| Desired Specifications: | ||||||||||||
| Critical gain margin ≥ 6 dB | ||||||||||||
| Critical phase margin ≥ 40 degrees | ||||||||||||
V. DESIGN ALGORITHM |
||||||||||||
| Step1: Considering ideal actuator system parametric expressions for GM, PM, GCF and PCF of autopilot and simple relations of Kp and Kq are derived. | ||||||||||||
| Step2: Next autopilot in yaw plane has been analyzed deriving expressions GM, PM, GCF and PCF implementing the actuator dynamics (ωa, ζa) in parametric form. | ||||||||||||
| Step3: Loss in GM and PM value in presence of a given actuator with a desired specifications GM ≤ 6 dB and PM ≤ 40 degree have been utilized. | ||||||||||||
| Step4: Set a value of PM as PMe = PMd +PMd/2. PMd is the desired PM. | ||||||||||||
| Step5: Find out new GM, PM, GCF and PCF. | ||||||||||||
| Step6: Check whether new PM is greater than or equal to PMd. If new PM is less than PMd then go to Step7, otherwise go to Step4. | ||||||||||||
| Step7: Set PMe1 =PMe -1 and go to Step 5. | ||||||||||||
| Step8: Print the values of Kp and Kq. | ||||||||||||
VI. NATURE OF BODY RATE, FIN DEFLECTION, FIN DEFLECTION RATE FOR CONTROL GAINS |
||||||||||||
VII. CONCLUSION |
||||||||||||
| A simple design approach has been developed for designing two-loop missile autopilot in yaw plane In this work modeling of two-loop autopilot has been developed for tail-controlled system. Simple expressions in parametric form have been derived to find out the GM, PM, GCF and PCF. It was observed that this methodology gives results comparable to what had been obtained by G.Das et al in[2], but this approach of evaluation of control gains is comparatively simpler and requires less computational efforts. The proposed approach may be advantageously used to find out whether a combination of specified phase margin and gain margin pairs could be obtained for a given aerodynamic data set and actuator dynamics with the twoloop autopilot configuration. In the future this work may be extended, by taking system nonlinearities into consideration. | ||||||||||||
ACKNOWLEDGMENT |
||||||||||||
| We extend our sincere gratitude to Prof. S.K. Goswami, EE Dept., Jadavpur University and faculty members of Bankura Unnayani Institute of Engineering for providing us the technical assistance for carrying out the necessary experiments of our research work. | ||||||||||||
Tables at a glance |
||||||||||||
|
||||||||||||
Figures at a glance |
||||||||||||
|
||||||||||||
References |
||||||||||||
|