Designing of TOU Power Price Based on
Game-Theoretic Approach

K.R.Akshara; Prof. P.Sangameshwara Raju

Designing of TOU Power Price Based on Game-Theoretic Approach

K.R.Akshara, Prof. P.Sangameshwara Raju

Department of EEE, SV University, Tirupati, AP, India
Department of EEE, SV University, Tirupati, AP, India

Related article at Pubmed, Scholar Google

Visit for more related articles at International Journal of Advanced Research in Electrical, Electronics and Instrumentation Engineering

Abstract

Requirement for power differs throughout the day, improving the average cost of power source. A timeof- use (TOU) expense has been suggested as a demand-side management (DSM) technique to influence customer requirements. In this paper, we explain a game-theoretic approach to improve TOU expenses strategies (GT-TOU). We recommend designs of expenses to power organizations coming up from customer demand fluctuations, and designs of customer fulfillment with the difference between the affordable demand and the actual intake.

Keywords

Electricity price, game theory, optimization, smart grid, time-of-use.

INTRODUCTION

The fluctuation of energy demand throughout the day has long been a problem for energy companies. During peak time, the energy companies encounter significant pressure to provide clients with enough h energy, and may even have to ration the energy resource of certain locations when the gap between demand and development is too large. During off-peak time, only a few of generators are needed to offer sufficient electricity to meet up with client need, and the nonproductive generators result in a invest of development prospective. The system complete is not the highest possible complete that a system can offer, but operate far from base load is not cost efficient, and may harm the stability of the Time-of-use (TOU) costs is an efficient technique of demand-side management (DSM) that application companies can implement to influence customer actions. Hart way et al. confirmed experimentally that TOU is profitable to a application organization, and that in common, the customers are satisfied with the TOU cost choice. California’s Statewide Pricing Lead revealed that personal and small-to-medium commercial and professional clients are willing to reduce their peak-period power use due to time-varying pricing.

GAME MODEL FOR SINGLE USER TYPE

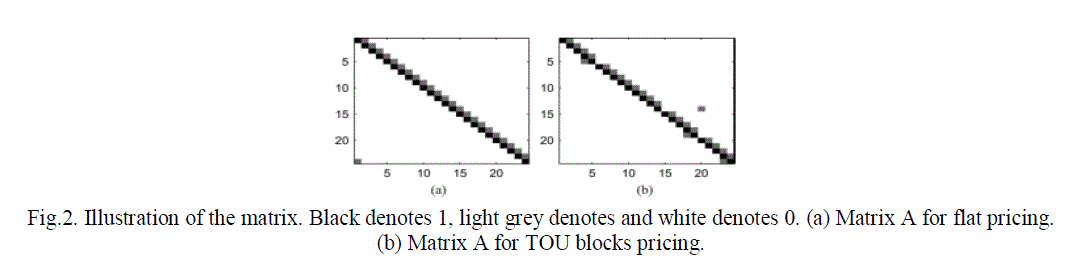
In this part we prepare the representation with a single type of user. We divide a day into N periods, where depends on the situation of the application. For hourly-based pricing, the notations are planned below.

Ck: marginal cost of electricity

pk: unit sales price of electricity

gk: electricity generation

dk: nominal user demand

lk: actual user load in response to the price

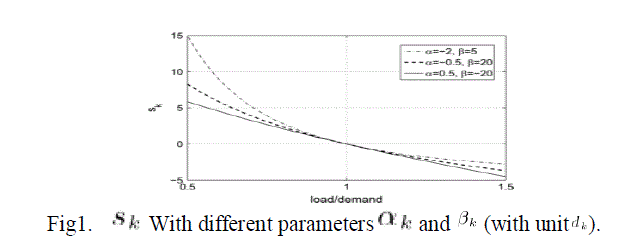
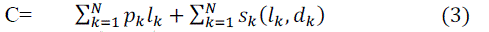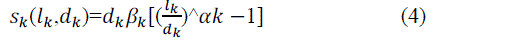III. MODEL WITH MULTIPLE USER TYPES

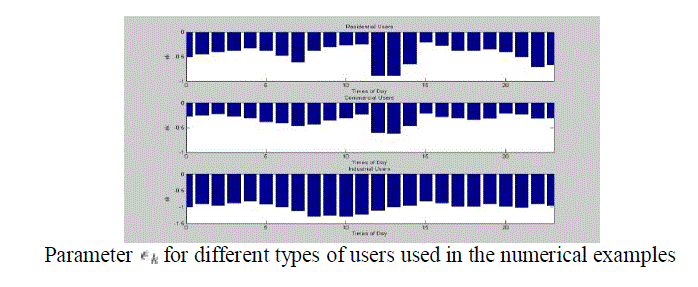
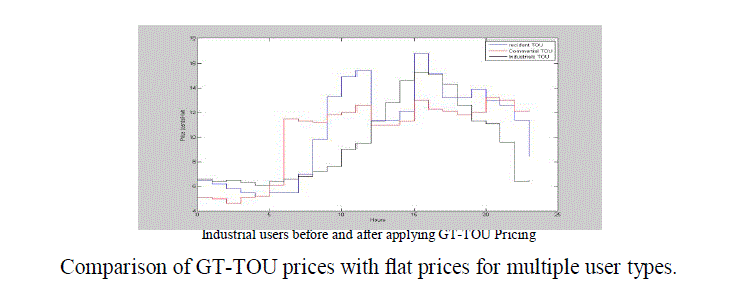
We consider three types of users: residential users (R), commercial users (B), and small industrial users (F). These users have different price response characteristics

Residential users:

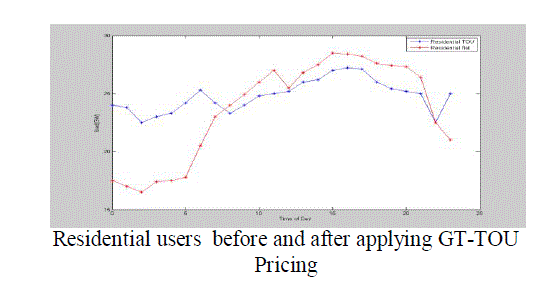
Users in places are usually delicate to price change, and they would like to modify their intake of electricity according to the time-varying costs. The flexibility of residential customers is relatively low, as they have limited capability to decrease or improve their complete use of power.

Commercial users:

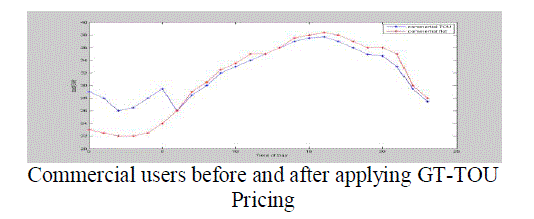
During office time, the requirements for power in business districts are great, and professional customers do not want to decrease the use of power which may impact their business. Power preservation techniques, however, can be used to preserve power if the power cost is great. Aspect of the less time-urgent perform can also be planned to other times of day.

Industrial users:

Industrial users: Commercial customers, especially those with great power consumption facilities, use a lot of power. The objective is to stage the complete load of all customers instead of just one type of customer. The organization and each kind of customer would have a utility function reflecting its overall profit/cost. The application functions are detailed below, where u1 is for the application company u2R,u2B and u2F are for personal, professional, and professional customers, respectively:

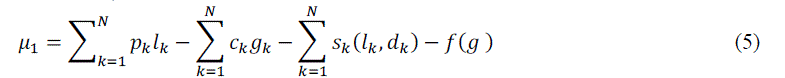commitment problem in the day-ahead market and an economic dispatch model in the real-time market. Other settings include information exchange, cooperation, and use of forecasting capabilities by the suppliers

V. CONCLUSIONS

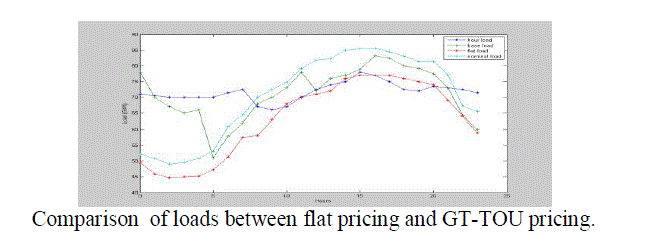
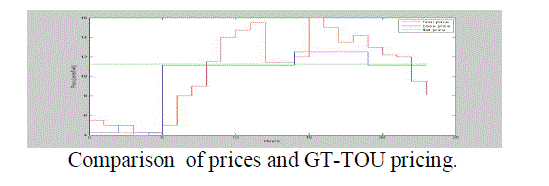
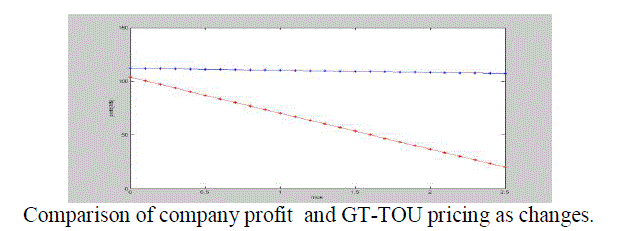
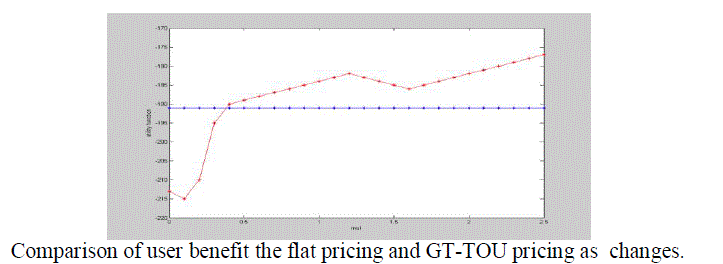
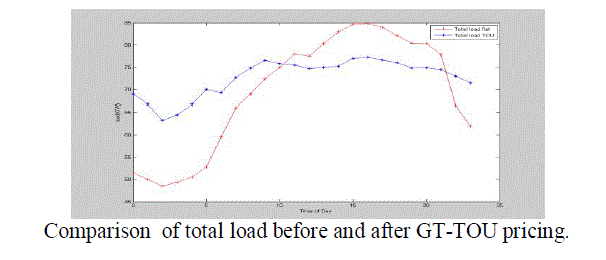
We suggested a maximum game-theoretic TOU electricity pricing technique (GT-TOU). We developed application functions for both power organizations and customers, and fixed for a Nash equilibrium, which provides maximum costs and customer reactions. The pricing strategy is flexible, as the design is appropriate for several pricing patterns, such as on per hour basis costs and time-block TOU costs. Simulation results illustrate that our approach can level user demand, raise the profits of the service companies, and decrease component prices for electrical energy users, and make sure overall user benefit. The leveled user load also potentially helps make sure a more steady power system.

References

C. W. Gellings, “The concept of demand-side management for electric utilities,” Proc. IEEE, vol. 73, pp. 1468–1470, 1985.
S.Rahman and SR.Rinaldy, “Anefficient load model for analyzing demand side management impacts,” IEEE Trans. Powe rSyst.,vol.8, pp. 1219– 1226, 1993.
M. Fahrioglu and F. L. Alvarado, “Designing cost effective demand management contracts using game theory,” in Proc .IEEEPower Engineering Society 1999 Winter Meeting, New York, Jan. 1999, pp.427–432.
M. Fahrioglu and F. L. Alvarado, “Designing incentive compatible contracts for effective demand management,” IEE E Trans. Power Syst.,vol. 15, pp. 1255–1260, 2000.
D.W.CavesandL.R.Christensen,“Econometric analysis of residential time-of-use electricity pricing experiments,” J. Econometr., vol.14, pp. 287– 306, 1980.