ISSN ONLINE(2278-8875) PRINT (2320-3765)
ISSN ONLINE(2278-8875) PRINT (2320-3765)
Chandrpal singh1, Abhaskumar singh2,Priyeshkumar pandey3,Harmendra Singh4
|
Visit for more related articles at International Journal of Advanced Research in Electrical, Electronics and Instrumentation Engineering
Transmission loss and Fair allocation of deregulated market participants is essential in the present restructured electricity markets.This paper proposes a direct method to find the loss allocation. The implementation of market mechanisms to remunerate distributed generation should take into account a non-discriminatory access to distribution networks. In consequence, power losses of distribution network must be fairly allocated among the all distributed generators and consumers. Several methods for power loss cost allocation have been proposed in the literature, divided basically into two groups. Firstly, methods as postage stamp, mw-mile, circuit based and proportional sharing have been supported on an arbitrary allocation of power losses between consumers and generators. The methodology is based on simple circuit laws and does not involve any assumptions. Considering the real power injection and real power loss contribution factors loss allocation can be done. Case study of the proposed loss allocation methodology is conducted on 2 bus and 6 bus system.
Keywords |
| Load flow, Transmission pricing, Pro-rata and Proportional Sharing Method, Transmission Loss Allocation. |
INTRODUCTION |
| Transmission loss allocation is important in restructured electricity markets. Since generators and demands are all connected to the same network, actions by one participant can have significant effects on others making it difficult to investigate the cost, each participant is responsible for it. It is difficult to achieve an efficient transmission loss allocation scheme that could fit all market structures in different locations. The ongoing research on transmission pricing indicates that there is no generalized agreement on pricing methodology. In practice, each restructuring model has chosen a method that is based on a particular characteristic of its network. The most common and simplest approach reported in the literature for transmission loss is the so called postage – stamp method, depends only on the amount of power moved and the duration of its use, irrespective of the supply and delivery points, distance of transmission usage. A participant, who uses the transmission system lightly, i.e. at a shorter electrical distance, actually subsidizes others who use the system heavily.. In the flow based methods, J. Baileket al proposed a method for loss allocation, where in considering merit order approach generation dispatch and nodal clearing prices aredetermined initially neglecting transmission losses and later on loss allocation is done among generators and demands. The flow based methods use the proportional sharing principle, which implies that any active power flow leaving a bus is proportionally made up of the flows entering that bus, such that Kirchhoff’s current law is satisfied. For the loss allocation, the share of generators and demands must be specified such as 50% loss among generators and 50% loss among loads. Equivalent bilateral exchanges method proposed for loss allocation does not require the choice of an arbitrary slack bus and is flow based in the sense that the loss allocated to individual agents takes into account their relative network positions. |
| In this paper we calculate the transmission losses with the different methods and also compare them. We also calculated the transmission losses pricing. In this paper we discussed about two methods (1) proportional sharing method and (2) pro rata method. |
NETWORK LOSSES AND LOSS ALLOCATION METHOD |
| Generally, in real power system the total generation is always larger than the total load demand because the total generation is equal to the total load demand counting the losses. |
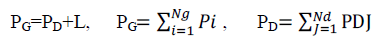 |
| Where, |
| PG= total active power generated. |
| PGI=power output of generator of bus i |
| PD=total active power demand |
| PDJ=active power demand by consumers of bus j |
| L= transmission power loss |
| NG=number of generator buses |
| ND=number of demand buses |
| Lately, although they are number of existing loss allocation methods this paper only focus on two method are, |
| 1 .Pro rata allocation. |
| 2. Proportional sharing allocation. |
(A) PRO RATA METOD |
| PR Procedures: First, losses are globally assigned to generators and consumers, for instance 50% of losses are allocated to each category. Then, a proportional allocation rule is used: the losses allocated to a generator (consumer) are proportional to its corresponding level of energy generation (consumption). A PR procedure is currently used in the electricity market of mainland Spain where 100% of losses are allocated to consumers. This principle is simple and easy to understand. The losses allocates to consumer proportionally with the level of energy consumption. |
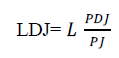 |
| This equation represents the pro rata allocation of losses to the load at bus j. PD is the total real power consumed and PDjis the real power consumed by the loads of bus j. while LDJ is the losses allocated at the demand j. the transmission loss is charged to the consumers through uniform pro rata charge. Uniform means that the same bid for each hour is being submitted.Pro rataprocedures are simple to understand and implement. However, they “ignore” the network. That is, two identical demands located respectively near generating buses and far away from these buses are equally treated, and this is unfair for the load located near the generating buses. |
(B)PROPORTIONAL SHARING METOD |
| Proportional Sharing Procedures: The use of the results of a converged power flow plus a linear proportional sharing principle make it possible for the allocation of losses to generators and consumers. This principle states that “the power flow reaching a bus from any power line splits among the lines evacuating power from the bus proportionally to their corresponding power flows,” which is neither provable nor disprovable. Proportional sharing procedures, on top of electrical laws, require the assumption of the proportional sharing principle. Using this principle, losses are allocated by linear procedures. To allocate losses to demands, the method relies on a simple principle: losses associated with every line whose flow enters a given bus are transferred to the lines whose flows leave the bus (or demands in that bus) proportionally to the flows of those lines (the flows of which leave the bus). It should be noted that a systematic application of this principle originates that all losses are allocated to demands. Analogously, in order to allocate losses to generators, the method relies on a simple principle: losses associated with every line whose flow leaves a given bus are transferred to the lines whose flows enter the bus (or generations in that bus) proportionally to the flows of those lines (whose flows enter the bus). |
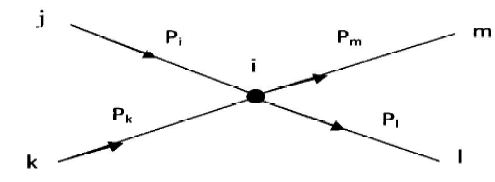 |
| Fig:1 principle of proportional sharing |
| According to figure 1, there are 4 branches connected with node i where j and k are the inflow branches while m and l are the outflow branches. By proportional sharing principle, the power at line:- |
 |
| To understand the allocation method, it can be considered by tracing gross flow to the load from generator sources. The formula of the tracing by gross flows are same with upstream tracing algorithm but the system is assuming feed with the actual generation in a lossless system. The power equation at node i considering the power inflows from upstreamlooking algorithm is define by, |
 |
 |
| Pg=unknown vector of gross nodal flows |
| Au= upstream distribution matrix |
| According to matrix form, the equation (2) rearranges and becomes, |
 |
| Once the gross nodal flows have been determine, the gross line flows and gross demands can be found by using the proportional sharing principle. To allocate losses to demand, this method relies on a simple principle: losses associated with every line whose flows enters a given bus are transferred to the lines. Whose flow leave the bus proportionally to the flows of those line. The gross flow in line i-l is |
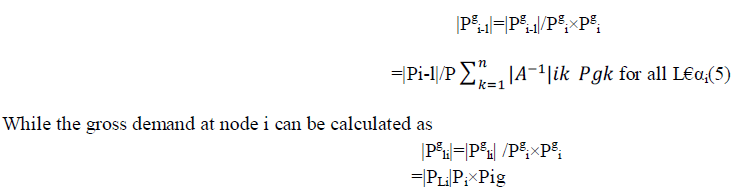 |
| Hence the difference between the gross demand and the actual demand is, |
| Where delta Pli gives the loss atteched by power flowing from the generator to particular load. Therefore, by the upstream-looking algorithm,contribution of each generator to the load can be determined. |
| Due to the fact that no unique or ideal procedure exists, any loss allocation algorithm should have most of the desirable properties stated below: |
| 1) To be consistent with the results of a power flow; |
| 2) To depend on the amount of energy either produced |
| 3) To depend on the relative location in the transmission network; |
| 4) To avoid volatility; |
| 5) To provide appropriate economic marginal signals; |
| 6) To be easy to understand; |
| 7) To be simple to implement. |
RESULTS |
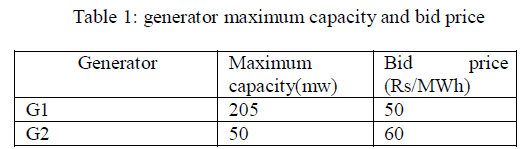
| A simple bus network with 2 buses such as shown in figure ,is used to compare 2 different method to investigate the impact of different loss allocation methods. This network is considerd to be operating in the pool system. |
 |
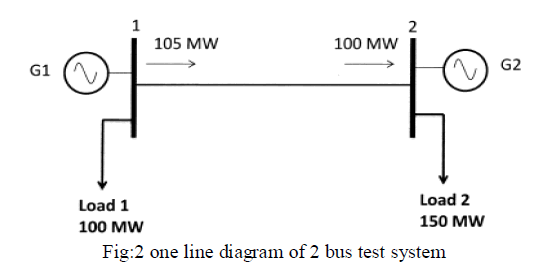 |
| Loads 1 and 2 are 100MW and 150MW respectively and the transmission loss is assumed to be 5MW. The transmission system is considered as not having a limit and constraint. The one line diagram shows that G1 and G2 generate up to its capacity as both loads 1 and 2 draw power from it. The export from G1to load 2 is 105MWincluded losses. The desired balance of load 2 is only 50MWand is supplied by G2. It is known as the cost of transmission loss and the amount due to this loss is Rs 100/hr |
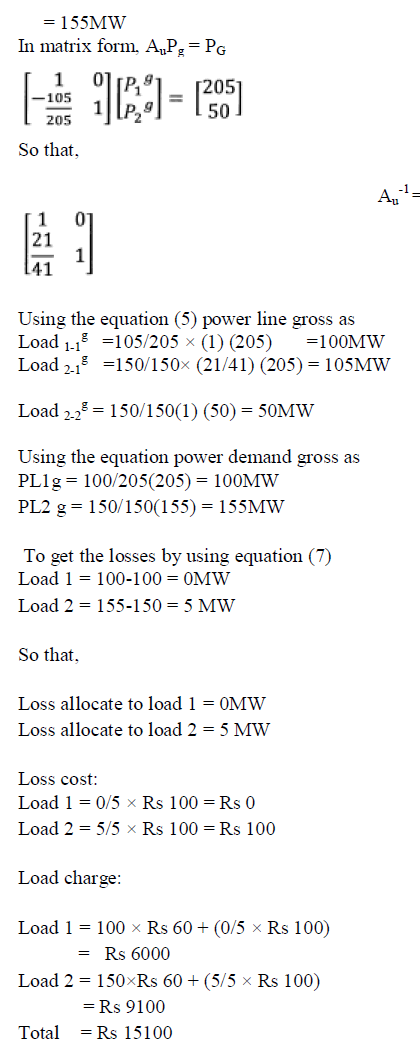
(A) PRO RATA METHOD |
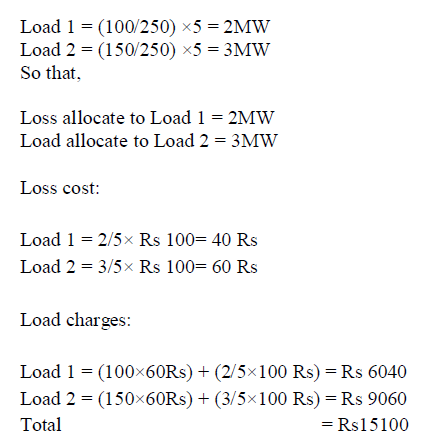
| Firstly the pro rata method is applied to the networking fig 2, using equation (1) the allocated for each load, |
 |
(B) PRAPORTIONAL SHARING METHOD |
| After that, this 2 bus test system is applied with proportional sharing method. The network consist of two buses and it is easy to known that load2 attracts the losses. For the network system that has more than 2 buses, the electricity traced by the equation in discussed in upper section. |
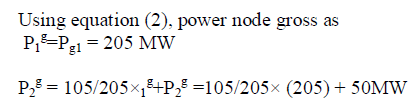 |
 |
(B) WORKED EXAMPLE |
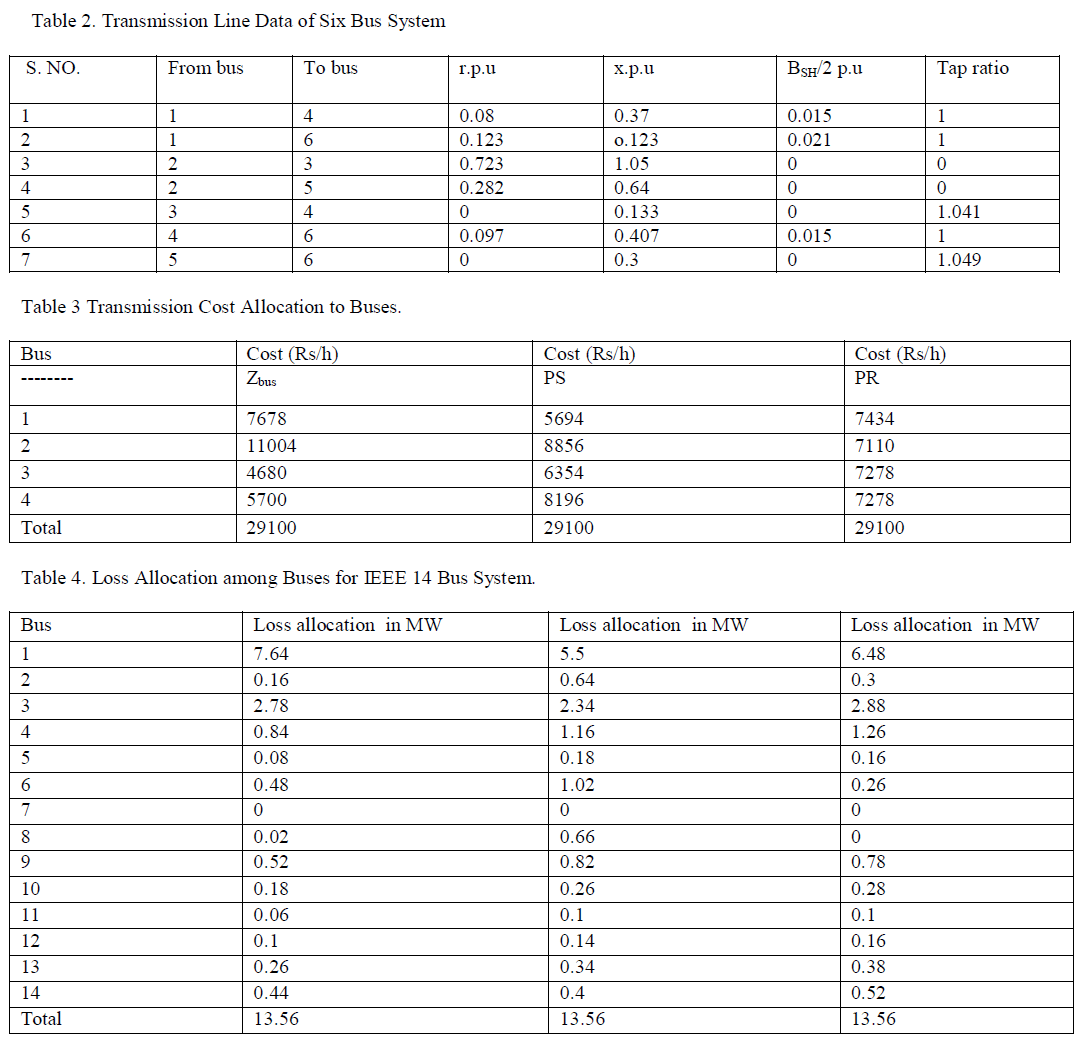
| The methodology is explained with the help of six bus system. Two generators (located at buses 1 and 2) sup-ply the power demand (located at buses 3, 5 and 6), while bus 4 is a zero injection (transfer) bus. The total real power loss in the system 8.37 MW is allocated among all 6 buses using the proposed method and its results are compared with those of the results obtained from existing methods in the literature. Base case bus voltages are obtained from N-R load flow solution for a six bus system whose line and bus data is shown in Tables 2.From close observation of the elements of Table2, it is noticed that the algebraic sum of all elements in any row, say ith row, gives the “real power injection” at that bus i.e. ithbus power injection. Here, positive sign indicates the power generation and negative sign indicates the power demand. Further, it is to be noted that algebraic sum of all elements in any column, say ith column, gives the real power loss in the transmission line corresponding to that column i.eith line. This table is useful for dual purposes i.e. transmission loss as well as cost allocations. It has been arrived at without any assumptions and the results are highly reliable. |
 |
CONCLUSIONS |
| The proposed loss allocation method has been tested on IEEE 14 bus test system and the losses are allocated among the buses. Results are compared with those of the results obtained using Z-bus loss allocation method, Pro-rata (PR) (considering currents and powers), Proportional sharing (PS), Test results of loss allocation among buses for the IEEE 14 bus system using the proposed method and the other methods are presented in table.In this paper, starting from a converged load flow solution, contribution factors of each complex power injection at a bus to the complex line loss in each of the transmission line are found out. At the same time, contributions of each of the transmission lines to the complex power injection at a bus are determined. These contributions allow allocation of loss among the buses based on the usage. Transmission cost for all of the system in every state is constant. This method because if use from load flow and true result in verity load can be used in smart grids and electricity market |
References |
|