ISSN: 2320-2459
ISSN: 2320-2459
Jeffery Bub*
Department of Philosophy, University of Maryland, Maryland, USA
Received: 01-Aug-2023, Manuscript No. JPAP-23- 108687; Editor assigned: 03- Aug-2023, Pre QC No. JPAP- 23-108687 (PQ); Reviewed: 17-Aug-2023, QC No. JPAP-23- 108687; Revised: 24-Aug- 2023, Manuscript No. JPAP- 23-108687 (R) Published: 31- Aug-2023,DOI:10.4172/2320- 2459.11.3.003.
Citation: Bub J. The Frauchiger-Renner Argument: A Critical Analysis. Res Rev J Pure Appl Phys. 2023;11:003.
Copyright: © 2023 Bub J. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited.
Visit for more related articles at Research & Reviews: Journal of Pure and Applied Physics
Can quantum theory model complex systems that include agents who are themselves using quantum theory? This question is answered by Frauchiger and Renner in their seminal paper “Quantum Theory Cannot Consistently Describe the use of Itself”.
Frauchiger and Renner propose a variant of the well-known Wigner’s Friend Gedankenexperiment modified by a construction by Hardy. In Wigner’s argument, the Friend, F, measures a qubit observable in an isolated laboratory containing the qubit, the measuring apparatus and F. Wigner, W, outside the
laboratory, is assumed to have the technological ability to measure arbitrary observables of the laboratory and its contents, including F and to perform arbitrary unitary operations on the laboratory as a complex quantum system. The Frauchiger-Renner variation involves a timed sequence of measurements by two agents, F and an additional Friend,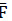 and two super agents, W and an additional Wigner,
and two super agents, W and an additional Wigner,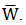 In addition to measurements, the agents make inferences about ‘certainty’ on the basis of the measurement outcomes according to two assumptions, Q and C, which are applied as inference rules
by the agents, and a third assumption, S, which prohibits inconsistent
inferences and is not invoked until the last step of the argument. The assumptions are straightforward.
In addition to measurements, the agents make inferences about ‘certainty’ on the basis of the measurement outcomes according to two assumptions, Q and C, which are applied as inference rules
by the agents, and a third assumption, S, which prohibits inconsistent
inferences and is not invoked until the last step of the argument. The assumptions are straightforward.
Assumption Q
If an agent A has established that a quantum system Q is in a state 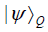 at time
at time 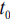 , and the Born probability of the outcome
, and the Born probability of the outcome  of a measurement of an observable X on Q in the state
of a measurement of an observable X on Q in the state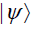 completed at time t is 1, then agent A can conclude: “I am certain that
completed at time t is 1, then agent A can conclude: “I am certain that 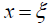 at time t”.
at time t”.
Assumption C
If an agent A has established: “I am certain that another agent 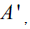 whose inferences about certainty are in accordance with Q, C, and S, is certain that
whose inferences about certainty are in accordance with Q, C, and S, is certain that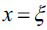 at time t”, then agent A can conclude: “I am certain that
at time t”, then agent A can conclude: “I am certain that 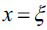 at time t”.
at time t”.
Assumption S
If an agent A has established “I am certain that 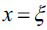 at time t”, then agent A cannot also establish “I am certain
that
at time t”, then agent A cannot also establish “I am certain
that 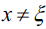 at time t”.
at time t”.
The argument shows that if agents, capable of performing measurements on quantum systems and making inferences on the basis of these measurements, are themselves modeled as quantum systems evolving unitarily in accordance with the assumptions Q, C, S, then, for the particular Gedankenexperiment considered, we end up with an inconsistency. The agents should be thought of as quantum computers programmed to carry out the sequence of measurements in the Gedanken experiment, and to draw inferences about “certainty” from measurement outcomes according to the assumptions Q and C constrained by the consistency requirement S.
Each stage of the experiment is characterized by a unitary evolution of the global quantum state in particular, the argument does not assume that the quantum state undergoes a “collapse” for observers inside a laboratory but not for outside observers. What might indeed require a collapse assumption is if agents were understood to always draw definite conclusions. But an agent’s inferences are physical processes. So an agent can draw conclusions in superposition, and an agent can be in a superposition of being certain and not being certain, or of being certain and drawing no conclusion. For further details, see [2].
The assumptions about certainty license time stamped entries in the memory registers of the agents. The experiment is conducted over many rounds and it turns out that for each round there is a finite probability, specifically 1/12, that W’s internal memory registers one of two possible measurement outcomes via the inference rules, as well as the direct observation of the alternative outcome. Assumption S prohibits conflicting entries with the same time stamp. So it seems that quantum mechanics cannot be applied to agents, as quantum systems, who are themselves measuring other quantum systems, if the agent’s actions are in accord with assumptions, Q, C, S. For some recent developments along these lines see [1-6].
[Crossref] [Google Scholar] [Pubmed]